Note: It is in the nature of book-writing that sometimes you write things that don’t end up appearing in the final book. I had a few such examples for Something Deeply Hidden, my book on quantum mechanics, Many-Worlds, and emergent spacetime. Most were small and nobody will really miss them, but I did feel bad about eliminating my discussion of the “delayed-choice quantum eraser,” an experiment that has caused no end of confusion. So here it is, presented in full. It’s a bit too technical for the book, I don’t know what I was thinking!
Let’s imagine you’re an undergraduate physics student, taking an experimental lab course, and your professor is in a particularly ornery mood. So she forces you to do a weird version of the double-slit experiment, explaining that this is something called the “delayed-choice quantum eraser.” You think you remember seeing a YouTube video about this once.
In the conventional double-slit, we send a beam of electrons through two slits and on toward a detecting screen. Each individual electron hits the screen and leaves a dot, but if we build up many such detections, we see an interference pattern of light and dark bands, because the wave function passing through the two slits interferes with itself. But if we also measure which slit each electron goes through, the interference pattern disappears, and we see a smoothed-out distribution at the screen. According to textbook quantum mechanics that’s because the wave function collapsed when we measured it at the slits; according to Many-Worlds it’s because the electron became entangled with the measurement apparatus, decoherence occurred as the apparatus became entangled with the environment, and the wave function branched into separate worlds, in each of which the electron only passes through one of the slits.
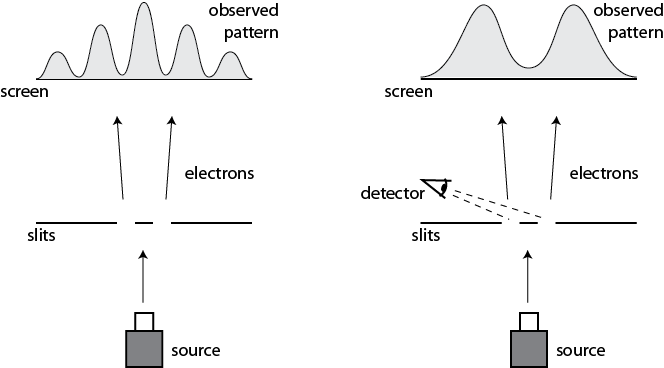
unless a detector measures which slit each electron goes through (right).
The new wrinkle is that we are still going to “measure” which slit the electron goes through, but instead of reading it out on a big macroscopic dial, we simply store that information in a single qubit. Say that for every “traveling” electron passing through the slits, we have a separate “recording” electron. The pair becomes entangled in the following way: if the traveling electron goes through the left slit, the recording electron is in a spin-up state (with respect to the vertical axis), and if the traveling electron goes through the right, the recording electron is spin-down. We end up with:
Ψ= (L)[↑] + (R)[↓].
Our professor, who is clearly in a bad mood, insists that we don’t actually measure the spin of our recording electrons, and we don’t even let them wander off and bump into other things in the room. We carefully trap them and preserve them, perhaps in a magnetic field.
What do we see at the screen when we do this with many electrons? A smoothed-out distribution with no interference pattern, of course. Interference can only happen when two things contribute to exactly the same wave function, and since the two paths for the traveling electrons are now entangled with the recording electrons, the left and right paths are distinguishable, so we don’t see any interference pattern. In this case it doesn’t matter that we didn’t have honest decoherence; it just matters that the traveling electrons were entangled with the recording electrons. Entanglement of any sort kills interference.
Of course, we could measure the recording spin if we wanted to. If we measure it along the vertical axis, we will see either [↑] or [↓]. Referring back to the quantum state Ψ above, we see that this will put us in either a universe where the traveling electron went through the left slit, or one where it went through the right slit. At the end of the day, recording the positions of many such electrons when they hit the detection screen, we won’t see any interference.
Okay, says our somewhat sadistic professor, rubbing her hands together with villainous glee. Now let’s measure all of our recording spins, but this time measure them along the horizontal axis instead of the vertical one. As we saw in Chapter Four, there’s a relationship between the horizontal and vertical spin states; we can write
[↑] = [→] + [←] ,
[↓] = [→] – [←].
(To keep our notation simple we’re ignoring various factors of the square root of two.) So the state before we do such a measurement is
Ψ = (L)[→] + (L)[←] + (R)[→] – (R)[←]
= (L + R)[→] + (L – R)[←].
When we measured the recording spin in the vertical direction, the result we obtained was entangled with a definite path for the traveling electron: [↑] was entangled with (L), and [↓] was entangled with (R). So by performing that measurement, we knew that the electron had traveled through one slit or the other. But now when we measure the recording spin along the horizontal axis, that’s no longer true. After we do each measurement, we are again in a branch of the wave function where the traveling electron passes through both slits. If we measured spin-left, the traveling electron passing through the right slit picks up a minus sign in its contribution to the wave function, but that’s just math.
By choosing to do our measurement in this way, we have erased the information about which slit the electron went through. This is therefore known as a “quantum eraser experiment.” This erasure doesn’t affect the overall distribution of flashes on the detector screen. It remains smooth and interference-free.
But we not only have the overall distribution of electrons hitting the detector screen; for each impact we know whether the recording electron was measured as spin-left or spin- right. So, instructs our professor with a flourish, let’s go to our computers and separate the flashes on the detector screen into these two groups — those that are associated with spin- left recording electrons, and those that are associated with spin-right. What do we see now?
Interestingly, the interference pattern reappears. The traveling electrons associated with spin-left recording electrons form an interference pattern, as do the ones associated with spin-right. (Remember that we don’t see the pattern all at once, it appears gradually as we detect many individual flashes.) But the two interference patterns are slightly shifted from each other, so that the peaks in one match up with the valleys in the other. There was secretly interference hidden in what initially looked like a featureless smudge.
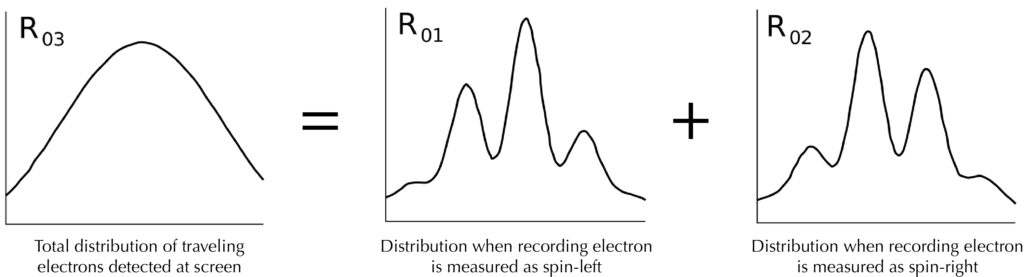
In retrospect this isn’t that surprising. From looking at how our quantum state Ψ was written with respect to the spin-left and -right recording electrons, each measurement was entangled with a traveling electron going through both slits, so of course it could interfere. And that innocent-seeming minus sign shifted one of the patterns just a bit, so that when combined together the two patterns could add up to a smooth distribution.
You professor seems more amazed by this than you are. “Don’t you see,” she exclaims excitedly. “If we didn’t measure the recording photons at all, or if we measured them along the vertical axis, there was no interference anywhere. But if we measured them along the horizontal axis, there secretly was interference, which we could discover by separating out what happens at the screen when the recording spin was left or right.”
You and your classmates nod their heads, cautiously but with some degree of confusion.
“Think about what that means! The choice about whether to measure our recording spins vertically or horizontally could have been made long after the traveling photons splashed on the recording screen. As long as we stored our recording spins carefully and protected them from becoming entangled with the environment, we could have delayed that choice until years later.”
Sure, the class mumbles to themselves. That sounds right.
“But interference only happens when the traveling electron goes through both slits, and the smooth distribution happens when it goes through only one slit. That decision — go through both slits, or just through one — happens long before we measure the recording electrons! So obviously, our choice to measure them horizontally rather than vertically had to send a signal backward in time to tell the traveling electrons to go through both slits rather than just one!”
After a short, befuddled pause, the class erupts with objections. Decisions? Backwards in time? What are we talking about? The electron doesn’t make a choice to travel through one slit or the other. Its wave function (and that of whatever it’s entangled with) evolves according to the Schrödinger equation, just like always. The electron doesn’t make choices, it unambiguously goes through both slits, but it becomes entangled along the way. By measuring the recording photons along different directions, we can pick out different parts of that entangled wave function, some of which exhibit interference and others do not. Nothing really went backwards in time. It’s kind of a cool result, but it’s not like we’re building a frickin’ time machine here.
You and your classmates are right. Your instructor has gotten a little carried away. There’s a temptation, reinforced by the Copenhagen interpretation, to think of an electron as something “with both wave-like and particle-like properties.” If we give into that temptation, it’s a short journey to thinking that the electron must behave in either a wave- like way or a particle-like way when it passes through the slits, and in any given experiment it will be one or the other. And from there, the delayed-choice experiment does indeed tend to suggest that information had to go backwards in time to help the electron make its decision. And, to be honest, there is a tradition in popular treatments of quantum mechanics to make things seem as mysterious as possible. Suggesting that time travel might be involved somehow is just throwing gasoline on the fire.
All of these temptations should be resisted. The electron is simply part of the wave function of the universe. It doesn’t make choices about whether to be wave-like or particle-like. But a number of serious researchers in quantum foundations really do take the delayed-choice quantum eraser and analogous experiments (which have been successfully performed, by the way) as evidence of retrocausality in nature — signals traveling backwards in time to influence the past. A form of this experiment was originally proposed by none other than John Wheeler, who envisioned a set of telescopes placed on the opposite side of the screen from the slits, which could detect which slit the electrons went through long after they had passed through. Unlike some later commentators, Wheeler didn’t go so far as to suggest retrocausality, and knew better than to insist that an electron is either a particle or a wave at all times.
There’s no need to invoke retrocausality to explain the delayed-choice experiment. To an Everettian, the result makes perfect sense without anything traveling backwards in time. The trickiness relies on the fact that by becoming entangled with a single recording spin rather than with the environment and its zillions of particles, the traveling electrons only became kind-of decohered. With just a single particle to worry about observing, we are allowed to contemplate measuring it in different ways. If, as in the conventional double- slit setup, we measured the slit through which the traveling electron went via a macroscopic pointing device, we would have had no choice about what was being observed. True decoherence takes a tiny quantum entanglement and amplifies it, effectively irreversibly, into the environment. In that sense the delayed-choice quantum eraser is a useful thought experiment to contemplate the role of decoherence and the environment in measurement.
But alas, not everyone is an Everettian. In some other versions of quantum mechanics, wave functions really do collapse, not just the apparent collapse that decoherence provides us with in Many-Worlds. In a true collapse theory like GRW, the process of wave- function collapse is asymmetric in time; wave functions collapse, but they don’t un- collapse. If you have collapsing wave functions, but for some reason also want to maintain an overall time-symmetry to the fundamental laws of physics, you can convince yourself that retrocausality needs to be part of the story.
Or you can accept the smooth evolution of the wave function, with branching rather than collapses, and maintain time-symmetry of the underlying equations without requiring backwards-propagating signals or electrons that can’t make up their mind.
Any interpretation that honors the mathematics should be taken seriously.
Regarding many worlds, it kind of reminds me of working with signal processing. We can represent
filters and signals in the time domain or we can represent them in the frequency domain.
If we only had the frequency domain representation but we did not know anything about the Fourier transform, we would create weird rules to try to infer the time domain representation from the frequency domain representation. We would make up ad hoc explanations of when to use and when not to use negative frequencies, and the like. People would get tied into philosophical knots trying to justify those notions.
Such a situation existed with Fermat’s principle of least time—the notion that a ray of light follows the path of minimum travel time through a medium. This led to speculations about how the light knew the minimum travel time path in advance. One was a teleological explanation. However, people who didn’t believe in teleology created a “pilot wave” notion–that there was a wave that led the way and like a scout found the minimum travel time path. It seemed that the classical aether theory, based on elastic wave theory, fit the bill. The faster compressional elastic waves were the “pilot” wave and the slower transverse shear waves were the electromagnetic waves.
Of course, the real answer is that rays are actually an approximate representation of wave propagation and that these minimum travel time paths are the “stationary” paths where the phase function of the waves is changing most slowly.
I find myself on a portion of the wave function that has not (yet) read your new book, so I’m confused about what I would observe in the following situation:
I use an apparatus to conduct the double-slit experiment without measuring/observing the electrons’ paths. But unknown to me, the apparatus contains a device that automatically recorded the electrons’ paths. Will I see an interference pattern or not? Will the result I see depend on whether or not anyone ever discovers the recording at some time in the future?
I have been hoping that you would write up this explanation, ever since you hinted that it was easy.
Thank you kindly,
This is an immensely helpful way to describe the Delayed choice Quantum erasure experiment. This shows how Everett eliminates the muddied thinking of Copenhagen
I love that you are tackling this subject, but this just raises even more questions. I am still very confused by all of this, (and I am sure I am in good company). So are you saying that it is entanglement with the measurement device that collapses the wave function? Or that the wave function still exists only we can no longer see it because our act of measurement collapsed our perception? So like with MWI there are many worlds but we can only perceive one? So in one sense the interference pattern is always there, only when we look, we can no longer see it?
So what dictates where we land when the wave function seemingly collapses?
Because if it is simply the idea that entanglement collapses the wave function, why does that not happen with random particle interactions? What is unique about the particles used in the measurement device? Clearly I am missing something, or not?
The silliness in interpretations of the delayed choice experiment is particularly blatant if you tell the classical version ( from https://algassert.com/post/1720 ).
Alice and Bob are playing a game. Bob has a six-sided die, a two-sided coin, and a small empty box to put the coin in. He rolls the die, gets a result between 1 and 6, and places the coin in the box heads up if the result is even (otherwise tails up). He then writes down his die roll, and carefully hands the box to Alice.
Alice now has a choice. She may either a) just open the box or b) shake the box before opening it (randomizing the coin). She then writes down whether the coin was face up or face down, and also writes down whether or not she shook the box.
After repeating the above process many times, Alice and Bob compare notes. They notice something amazing: Alice’s choice seems to have affected Bob’s die rolls, even though Bob wrote down his results before Alice’s choice. When Alice shook the box the distribution of die rolls was flat, but when she didn’t the distribution was banded! (Conditioned on the unshook coin being heads, the die roll was always even.) Clearly there is some sort of retrocausal effect…
The mistake in reasoning being made in the quantum delayed choice eraser experiment is exactly the same as the mistake in reasoning being made in that last paragraph. It’s unnecessarily interpreting correlation as retrocausality.
So when an observable has two eigenvalues, how many worlds are there – two? Two million? A continuum? Does the number of worlds relate to the probability of an observation? If yes, please explicitly exhibit the decomposition of the wavefunction into the appropriate worlds. And if the probabilities don’t come from the number of worlds, please explain where they do come from.
“True decoherence takes a tiny quantum entanglement and amplifies it, effectively irreversibly, into the environment. In that sense, the delayed-choice quantum eraser is a useful thought experiment to contemplate the role of decoherence and the environment in measurement.”
Another way to approach the above may be to think in terms only of “measurement” (the term’s being unsatisfactory notwithstanding). Whereby:
1) Entanglement and measurement share the same physics.
2) Decoherence leads to the same result as in 1), regardless of what the process is called.
Whereupon:
We’re saying, when two entities interact in the environment, that interaction results in quantum acquiring classical characteristics. Or simply “quantum becomes classical”. Which is, after all, the outcome of any measurement.
Once so reduced, neither collapse nor splitting of the wavefunction needs to be invoked?
Thanks, Sean. People (especially quantum woomeisters and their acolytes) keep bringing it up as some sort of counterintuitive mysterious result of quantum mechanics, but all I remember from my introduction to it (via PBS Spacetime, wonderful channel btw) is that interference patterns appear if you pick the dots on the screen corresponding to one of the basis states.
And of course said woomeisters start pontificating about how your choice affects reality and blah blah blah, and I sit there confused about how they ever reached that conclusion.
I like the “part of the wave function of the universe” razor.
But, as the thread already noted, as long as the different quantum theories are consistent with all observations, we must delay our choice between them.
To wit: “There’s a temptation, reinforced by the Copenhagen interpretation, to think of an electron as something “with both wave-like and particle-like properties.” Maybe there is a pre-quantum field theory temptation, but I think the duality idea vanished a century ago. A razor of another kind, still leaving Copenhagen theory, MW theory et cetera on the chin (but relativity put pilot wave theory in the suds, I think).
This is intriguing: “In some other versions of quantum mechanics, wave functions really do collapse, not just the apparent collapse that decoherence provides us with in Many-Worlds. … wave functions collapse, but they don’t un- collapse. If you have collapsing wave functions, but for some reason also want to maintain an overall time-symmetry to the fundamental laws of physics, …” But, entropy – which decoherence reminds of anyway, transporting state information irreversibly to the environment I take it. Does the collapse process necessarily need to be microstate reversible? This seem to suggest it does not: “The trickiness relies on the fact that by becoming entangled with a single recording spin rather than with the environment and its zillions of particles, the traveling electrons only became kind-of decohered.”
This is a digital universe ie virtual reality, the speed of light is the delta t (refresh rate of the virtual reality). In a digital reality stuff is ONLY rendered when required. If you don’t look at results of quantum erasure experiments for months, and think there must be ‘a going back in time’ this is not correct, what results you view are only rendered there and then, they have not been sat on storage device for months.
Sean, thank you for this. Wherever I was learning physics at school or undergraduate level it’s been a case of learning a wrong theory and later being told that it was a lie and here’s the next one. I suspected quantum mechanics and wave particle duality was full of nonsense but the teachers and the textbooks were adamant. Put me off the subject honestly. This many words correction is the first time the theory looks reasonable and true. Maybe we can enjoy that for a few years before replacing with a theory of abstract quantum computing and emergent spacetime.
One point, I don’t like the notion of splitting because it suggests discrete counting and that feels mind boggling. Personally I prefer to think that the we’ve function is a continuous multi dimensional wave that includes all the possibilities, each of then with low amplitude. Decoherence then reduces the amplitude of some of the space to zero, leaving it looking like branching stands. But it’s a continuous effect, but discrete logic
I liked this blog post (via your email distribution list). I also read Wiki’s page “Delayed-Choice Quantum Eraser” and the history of actual experiments. Your post helped me understand your many-worlds stance better. It’s like a short story. Connecting interference, bookended entanglement, and decoherence.
It’s refreshing to see you use some visuals (tables and diagrams) in chapter one of your book to characterize the differences between classical and quantum physics. Maybe a visualization of an identical simple system for each set of rules? – using modern measuring apparatuses in each case.
Certainly the so-called measurement problem is important. Even more interesting for me is the evolution of physics from a mechanics of the time evolution of positions & velocities of macroscopic objects responding to “forces” to that of positions & momentum of objects responding to energy dynamics to that of energy wave functions which yield probabilistic measurements.
Personally I am not sure about the reality of the wave function – as ontic vs. epistemic.
I don’t understand. Does it have to be many worlds or GRW? Couldn’t the collapse be random but only in a relatively spontaneous sense? Meaning that retrocausality is an illusion caused by the nature of nonlocality. If all particles are measurable in relation to other particles, and experiment exclusions are only meant to simplfy, since you aren’t able to calculate all the relations of all particles with each other at any given time, you are left guessing as to whether any one wave collapse is random or not.
Why don’t we dispense with all this silliness, embrace determinism like a lover, and postulate that ALL branches, be they past, present, or future, were created at the big bang, and every particle is just following the path it was destined to follow?
Sam Hawk asks: “ Why don’t we (…) embrace determinism (…)?”
As if we had a choice…
Related:
The ‘Delayed Choice Quantum Eraser’ Neither Erases Nor Delays
https://arxiv.org/pdf/1905.03137.pdf
Why Delayed Choice Experiments Do Not Imply Retrocausality
http://www.ellerman.org/wp-content/uploads/2015/01/OnLineFirstReprint.pdf
Good point.
Maybe that’s one reason why quantum mechanics appears mysterious. Suppose quantum reality is non-deterministic, yet we keep expecting it to be deterministic (since we “have no choice”), then the result is … expected.
I am currently reading your book. I like MWI, but because, philosophically, I like eternalism I would like to see an eternalist interpretation. of MWI. This would eliminate the presentist splitting of the universe. Instead I think (or would like to think) there is a block Hilbert space universe in which the universal Schrodinger’s equation lives and the dynamic universe splitting is simply an unnecessary assumption.
What about if we imagine that a human observer is watching at the apparatus. She and only she knows which slit the electron passed through. While the electron flies towards the screen she goes to Australia or, perhaps, she dies. Another human observe the next electron and goes away for ever. And so on. After one million of observations, have we an interfererence pattern in the screen?
The problem with MWI is that the different branches don’t exist as a place you can ever go to. For example the past can be said to exist but you can’t visit it. The billionth prime number can be said to exist but you can neither find it with the Hubble space telescope or weigh it on a scale. Spider man can be said to exist as people are spending millions arguing over who owns it. So the whole “exists” thing strikes me as an empty semantic debate.
Luis says: “What about if we imagine that a human observer is watching at the apparatus.”
It depends. If she is in contact with the same thermodynamic background as you then you have effective wave collapse. She does not have to tell you anything. All you have to do is smell her perfume or see her in the distance. You don’t even have to be conscious of the interaction. If you inhale some co2 molecules that she exhaled. This is why quantum computers are so hard to build. If your quantum logic gates interact in even the most trivial way with the outside world then your quantum computer becomes a very expensive space heater.
The quantum state is observer dependent. Consider Schrödinger’s cat for example. The cat is in a box such that it does not interact with the outside world in even the most trivial way. So we must view the cat in a superposition of alive/dead. But what doe the cat think about this? It knows very well what its state is.
Sam Hawk asks: “ Why don’t we (…) embrace determinism (…)?”
Or we can embrace nondeterminism and so each quantum particle does a random walk through its possibility space. Or we can say that each particle splits and takes all possible paths.
All of these possibilities make the same predictions. Worse none of these possibilities make any predictions at all. They can be consistent with QM but they can be consistent with a classical looking theory as well. They aren’t theories at all but rather expressions of philosophical priors. As such they can be dangerous.
Craig Gidney says: “The silliness in interpretations of the delayed choice experiment…”
Yes but the classical version cannot reproduce some aspects of QM such as violations of Bell’s inequality. It is after all just a local hidden variable theory. This is why it is so tempting to think in terms of retro-causality. Sure you can and should push back against it but the fact is QM is weirdly and totally non-classical.
I agree with Neil Bruce’s point on eternalism. Presentism does not make sense i.m.o. If we assume presentism then one can still apply the time evolution operator to the present state and then interpret this as a superposition of the future branches. So, the future states already exist as scrambled versions of the present state making it futile to assume presentism.
Hello Sean,
I love Mindscape and I’ve really been enjoying Something Deeply Hidden. So much so that I’ve been discussing the book with physicists and enthusiasts as I read it.
Recently we’ve been arguing over a critique by Sabine Hossenfelder (http://backreaction.blogspot.com/2019/09/the-trouble-with-many-worlds.html) that seems to charge that the Many Worlds Interpretation (MWI) is guilty of smuggling in the collapse postulate and reintroducing the measurement problem. The charge is that MWI is doing something that violates the Schrodinger equation.
Suppose you perform the spin entangled experiment you describe in your book. Branching/decoherence occurs. Your copy looks at his device and finds that it reads “spin-up”. But now, or so the charge goes, your copy updates the probability to 1 that the state is spin-up and he represents the wave function in a “collapsed” state for future experiments. In other words, your copy has taken a linear combination of terms he knows represented the state in the parent world and discarded all but one of them and renormalized in a way that incompatible with the Schrodinger equation. Otherwise he won’t be able to predict the correct probabilities in future experiments.
I get the sense that that I’m missing something here, but it’s doing my head in. My apologies to you and Sabine if I’ve misconstrued your arguments in any way. If you have time, could you comment on this apparent problem? Thanks in advance!
“Once so reduced, neither collapse nor splitting of the wavefunction needs to be invoked?”
if so, wouldn’t reducing the particle interaction to classical inherently mean a wave function collapse?
if not, would you be postulating that superposition =/= wave function collapse, and with the wave function being constant through time the eigenvalues/degrees of freedom could be treated as fractals within the spacetime continuum so that we are able to represent a particle’s path as the oscillation of a non-orientable topology with varied frequency such that its spatial entanglement can not be mapped out with absolute accuracy by fourier analysis?
The quote “Once so reduced, neither the collapse nor splitting of a wavefunction needs be invoked?” cited by LQG came from a comment on September 21. What preceded the quote were, essentially:
1) In a general sense, the outcome of any measurement consists of quantum reality becoming classical reality.
2) When measurement is treated as any interaction between two entities in the universe (via entanglement), the wavefunction describing the measured entity does not need to either collapse nor split.
Rephrased, neither a Copenhagen description nor an Everettian description needs to be specified.
Why?
The description has been simplified to: Whenever two entities interact in the environment, their entanglement results in quantum reality becoming classical reality.
Everything else remains unchanged, including the wavefunction. But instead of remaining constant through time as LQG stated, the wavefunction evolves according to the Schrodinger equation, exactly as in any quantum mechanics interpretation.
Meaning, quantum reality evolves over time according to the Schrodinger equation, until the moment of interaction. Whereupon, whichever entity (playing the role of one) being measured switches from quantum reality to become classical reality.
The outcome is random/probabilistic, as it depends on the particular status of evolution of the wavefunction, at the moment of interaction.
What allows this “interpretation” is the notion that quantum reality cannot be the classical physical reality (https://quantumfrontiers.com/2018/11/25/theoretical-physics-has-not-gone-to-the-dogs/comment-page-1/#comment-117520). If preferred, one could say that the wavefunction is not ontic. But nor does it have to be epistemic. It’s simply “nonphysical”.
Will leave the rest to real philosophers. But this notion of a nonphysical wavefunction describing quantum reality could perhaps provide some common ground for different interpretations in quantum mechanics?
To wit, there’s no physical wavefunction to collapse nor to split. Nothing is physical (classical) until nonphysical quantum is measured.
Dear Dr. Carroll,
You have mentioned before that there are formal proofs out there saying that under certain assumptions, one should act the same in a multiverse if there are multiple copies of you as if there were only one copy. I am struggling to find the papers you are referring to. Could you reference one or two of your favorites?