The other day I was amused to find a quote from Einstein, in 1936, about how hard it would be to quantize gravity: “like an attempt to breathe in empty space.” Eight decades later, I think we can still agree that it’s hard.
So here is a possibility worth considering: rather than quantizing gravity, maybe we should try to gravitize quantum mechanics. Or, more accurately but less evocatively, “find gravity inside quantum mechanics.” Rather than starting with some essentially classical view of gravity and “quantizing” it, we might imagine starting with a quantum view of reality from the start, and find the ordinary three-dimensional space in which we live somehow emerging from quantum information. That’s the project that ChunJun (Charles) Cao, Spyridon (Spiros) Michalakis, and I take a few tentative steps toward in a new paper.
We human beings, even those who have been studying quantum mechanics for a long time, still think in terms of a classical concepts. Positions, momenta, particles, fields, space itself. Quantum mechanics tells a different story. The quantum state of the universe is not a collection of things distributed through space, but something called a wave function. The wave function gives us a way of calculating the outcomes of measurements: whenever we measure an observable quantity like the position or momentum or spin of a particle, the wave function has a value for every possible outcome, and the probability of obtaining that outcome is given by the wave function squared. Indeed, that’s typically how we construct wave functions in practice. Start with some classical-sounding notion like “the position of a particle” or “the amplitude of a field,” and to each possible value we attach a complex number. That complex number, squared, gives us the probability of observing the system with that observed value.
Mathematically, wave functions are elements of a mathematical structure called Hilbert space. That means they are vectors — we can add quantum states together (the origin of superpositions in quantum mechanics) and calculate the angle (“dot product”) between them. (We’re skipping over some technicalities here, especially regarding complex numbers — see e.g. The Theoretical Minimum for more.) The word “space” in “Hilbert space” doesn’t mean the good old three-dimensional space we walk through every day, or even the four-dimensional spacetime of relativity. It’s just math-speak for “a collection of things,” in this case “possible quantum states of the universe.”
Hilbert space is quite an abstract thing, which can seem at times pretty removed from the tangible phenomena of our everyday lives. This leads some people to wonder whether we need to supplement ordinary quantum mechanics by additional new variables, or alternatively to imagine that wave functions reflect our knowledge of the world, rather than being representations of reality. For purposes of this post I’ll take the straightforward view that quantum mechanics says that the real world is best described by a wave function, an element of Hilbert space, evolving through time. (Of course time could be emergent too … something for another day.)
Here’s the thing: we can construct a Hilbert space by starting with a classical idea like “all possible positions of a particle” and attaching a complex number to each value, obtaining a wave function. All the conceivable wave functions of that form constitute the Hilbert space we’re interested in. But we don’t have to do it that way. As Einstein might have said, God doesn’t do it that way. Once we make wave functions by quantizing some classical system, we have states that live in Hilbert space. At this point it essentially doesn’t matter where we came from; now we’re in Hilbert space and we’ve left our classical starting point behind. Indeed, it’s well-known that very different classical theories lead to the same theory when we quantize them, and likewise some quantum theories don’t have classical predecessors at all.
The real world simply is quantum-mechanical from the start; it’s not a quantization of some classical system. The universe is described by an element of Hilbert space. All of our usual classical notions should be derived from that, not the other way around. Even space itself. We think of the space through which we move as one of the most basic and irreducible constituents of the real world, but it might be better thought of as an approximate notion that emerges at large distances and low energies.
So here is the task we set for ourselves: start with a quantum state in Hilbert space. Not a random or generic state, admittedly; a particular kind of state. Divide Hilbert space up into pieces — technically, factors that we multiply together to make the whole space. Use quantum information — in particular, the amount of entanglement between different parts of the state, as measured by the mutual information — to define a “distance” between them. Parts that are highly entangled are considered to be nearby, while unentangled parts are far away. This gives us a graph, in which vertices are the different parts of Hilbert space, and the edges are weighted by the emergent distance between them.
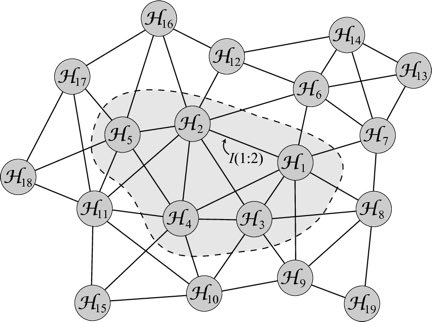
We can then ask two questions:
- When we zoom out, does the graph take on the geometry of a smooth, flat space with a fixed number of dimensions? (Answer: yes, when we put in the right kind of state to start with.)
- If we perturb the state a little bit, how does the emergent geometry change? (Answer: space curves in response to emergent mass/energy, in a way reminiscent of Einstein’s equation in general relativity.)
It’s that last bit that is most exciting, but also most speculative. The claim, in its most dramatic-sounding form, is that gravity (spacetime curvature caused by energy/momentum) isn’t hard to obtain in quantum mechanics — it’s automatic! Or at least, the most natural thing to expect. If geometry is defined by entanglement and quantum information, then perturbing the state (e.g. by adding energy) naturally changes that geometry. And if the model matches onto an emergent field theory at large distances, the most natural relationship between energy and curvature is given by Einstein’s equation. The optimistic view is that gravity just pops out effortlessly in the classical limit of an appropriate quantum system. But the devil is in the details, and there’s a long way to go before we can declare victory.
Here’s the abstract for our paper:
Space from Hilbert Space: Recovering Geometry from Bulk Entanglement
ChunJun Cao, Sean M. Carroll, Spyridon MichalakisWe examine how to construct a spatial manifold and its geometry from the entanglement structure of an abstract quantum state in Hilbert space. Given a decomposition of Hilbert space H into a tensor product of factors, we consider a class of “redundancy-constrained states” in H that generalize the area-law behavior for entanglement entropy usually found in condensed-matter systems with gapped local Hamiltonians. Using mutual information to define a distance measure on the graph, we employ classical multidimensional scaling to extract the best-fit spatial dimensionality of the emergent geometry. We then show that entanglement perturbations on such emergent geometries naturally give rise to local modifications of spatial curvature which obey a (spatial) analog of Einstein’s equation. The Hilbert space corresponding to a region of flat space is finite-dimensional and scales as the volume, though the entropy (and the maximum change thereof) scales like the area of the boundary. A version of the ER=EPR conjecture is recovered, in that perturbations that entangle distant parts of the emergent geometry generate a configuration that may be considered as a highly quantum wormhole.
Like almost any physics paper, we’re building on ideas that have come before. The idea that spacetime geometry is related to entanglement has become increasingly popular, although it’s mostly been explored in the holographic context of the AdS/CFT correspondence; here we’re working directly in the “bulk” region of space, not appealing to a faraway boundary. A related notion is the ER=EPR conjecture of Maldacena and Susskind, relating entanglement to wormholes. In some sense, we’re making this proposal a bit more specific, by giving a formula for distance as a function of entanglement. The relationship of geometry to energy comes from something called the Entanglement First Law, articulated by Faulkner et al., and used by Ted Jacobson in a version of entropic gravity. But as far as we know we’re the first to start directly from Hilbert space, rather than assuming classical variables, a boundary, or a background spacetime. (There’s an enormous amount of work that has been done in closely related areas, obviously, so I’d love to hear about anything in particular that we should know about.)
We’re quick to admit that what we’ve done here is extremely preliminary and conjectural. We don’t have a full theory of anything, and even what we do have involves a great deal of speculating and not yet enough rigorous calculating.
Most importantly, we’ve assumed that parts of Hilbert space that are highly entangled are also “nearby,” but we haven’t actually derived that fact. It’s certainly what should happen, according to our current understanding of quantum field theory. It might seem like entangled particles can be as far apart as you like, but the contribution of particles to the overall entanglement is almost completely negligible — it’s the quantum vacuum itself that carries almost all of the entanglement, and that’s how we derive our geometry.
But it remains to be seen whether this notion really matches what we think of as “distance.” To do that, it’s not sufficient to talk about space, we also need to talk about time, and how states evolve. That’s an obvious next step, but one we’ve just begun to think about. It raises a variety of intimidating questions. What is the appropriate Hamiltonian that actually generates time evolution? Is time fundamental and continuous, or emergent and discrete? Can we derive an emergent theory that includes not only curved space and time, but other quantum fields? Will those fields satisfy the relativistic condition of being invariant under Lorentz transformations? Will gravity, in particular, have propagating degrees of freedom corresponding to spin-2 gravitons? (And only one kind of graviton, coupled universally to energy-momentum?) Full employment for the immediate future.
Perhaps the most interesting and provocative feature of what we’ve done is that we start from an assumption that the degrees of freedom corresponding to any particular region of space are described by a finite-dimensional Hilbert space. In some sense this is natural, as it follows from the Bekenstein bound (on the total entropy that can fit in a region) or the holographic principle (which limits degrees of freedom by the area of the boundary of their region). But on the other hand, it’s completely contrary to what we’re used to thinking about from quantum field theory, which generally assumes that the number of degrees of freedom in any region of space is infinitely big, corresponding to an infinite-dimensional Hilbert space. (By itself that’s not so worrisome; a single simple harmonic oscillator is described by an infinite-dimensional Hilbert space, just because its energy can be arbitrarily large.) People like Jacobson and Seth Lloyd have argued, on pretty general grounds, that any theory with gravity will locally be described by finite-dimensional Hilbert spaces.
That’s a big deal, if true, and I don’t think we physicists have really absorbed the consequences of the idea as yet. Field theory is embedded in how we think about the world; all of the notorious infinities of particle physics that we work so hard to renormalize away owe their existence to the fact that there are an infinite number of degrees of freedom. A finite-dimensional Hilbert space describes a very different world indeed. In many ways, it’s a much simpler world — one that should be easier to understand. We shall see.
Part of me thinks that a picture along these lines — geometry emerging from quantum information, obeying a version of Einstein’s equation in the classical limit — pretty much has to be true, if you believe (1) regions of space have a finite number of degrees of freedom, and (2) the world is described by a wave function in Hilbert space. Those are fairly reasonable postulates, all by themselves, but of course there could be any number of twists and turns to get where we want to go, if indeed it’s possible. Personally I think the prospects are exciting, and I’m eager to see where these ideas lead us.
Are you aware of Thanu Padmanabhan’s work on a similar topic?
http://arxiv.org/abs/1603.08658
http://arxiv.org/abs/1512.06546
http://arxiv.org/abs/1508.06286
http://arxiv.org/abs/1505.05297
http://arxiv.org/abs/1410.6285
Paddy has to be one of the most interesting physicists, and one of the smartest, alive today. How can one person know so much, and still be a nice guy as well?
Although I would classify it as very advanced for a popular book, some here might enjoy reading his collection of essays on interesting physical topics, often involving relations between areas of physics and mathematics which are probably not obvious to most people. One of the themes is to start with a quantum description then derive the classical limit, rather than quantizing some classical system.
The link is to the proof of a review I wrote. It contains a small error, which was corrected in the published version. Bonus points if you can spot it.
we deal with complex numbers and pauli algebra – but there ought to be an elephant in the room – namely octonion algebra –
but we do not see this elephant – at least the Standard Model does not see it. The question is whether it would be needed to
make sense of the connections of space and particles at deeper levels than we can model at present. One expects a connection
just because complex octonions contain Pauli algebra ! How can anyone ignore that ? Unfortunately a lot of the literature seems
to go nowhere, eg octonion hilbert space.
It is possibly alarming that the Standard Model merrily ignores dark matter ( and dark anti-matter ) Are there 3 generations
of dark particles ? And does it fit the Yang Mills paradigm ? Maybe emergence of space is problematic without knowing more
about this stuff.
Sean,
Here’s a wild idea. Can there be a black hole effect? Just as radio waves only work at lower frequencies when transmitted under water or inside the earth, can gravitation simply be an extremely low frequency wave of electromagnetism when matter is extremely dense and massive?
Sean,
I have read this post carefully and find your approach bears a striking resemblence to a Loop Quantum Gravity paper “Quest for Quantum Gravity” using an approach they call Causal Dynamical Triangulation which seems similar to your node network. Their paper is: arXiv:1004.0352v1 [hep-th] 2 Apr 2010. In the abstract they find many of the emergent things that you have with the added advantage of Background Independence which is unlikely to emerge if a fixed background is assumed at the start.
If you were unaware of this approach, please have a look. If you were aware, please forgive me for the suggestion. I am not a theorist but a retired Marine Geophysicist and realize that the ‘Devil is in the details’ having followed the rapid advances in Astrophysics and Cosmology over the last 15 years.
Thanks you for having me in your distribution list- I look forward to every post.
Dave Prentiss
As far as I know from a couple of decades ago, it is not known how far entanglement can spread its influence. But, I have hypothesized a lot about it since then… From my own interpretation of quantum mechanics, the actual distance could be infinite for a particle traveling the speed of light. If you assume that quantum superposition stems from the frame of reference of light, then a particle traveling the speed of light would see itself in every position along its worldline. For a particle traveling less than the speed of light, that distance could be limited. Then I believe that it is possible for the distance for entanglement for an object traveling the speed of light to be infinite and possibly Lorentz Invariant, but an object traveling less than the speed of light would not be Lorentz Invariant. If Lorentz Invariance is an issue here, I would stick to particles that travel the speed of light… Or, I wouldn’t give up if it doesn’t work for particles that travel slower than the speed of light.
Hi Sean, If I correctly understand you, I appreciate your indication that we cannot measure spacetime with classical values. I suppose this line of thought began with Bell’s theorem. We can measure spacetime with probability distributions instead of classical values. Have you seen this 2014 review in CURRENT SCIENCE 107:2 (http://www.currentscience.ac.in/Volumes/107/02/0203.pdf)? Does this paper look relevant to your most recent paper? Peace, Jim
for views of the fundamental nature of discrete space-time from which fields and perhaps even quantum mechanics emerge , you might want to look at two papers:
“What is spacetime, really?” by Wolfram at http://blog.stephenwolfram.com/2015/12/what-is-spacetime-really/
“The Other Einstein: Einstein Contra Field Theory” by Stachel at Science in Context, 6(1), 275-290 (1993).
also, , item D in the General Remarks section that concludes Einstein’s “The Meaning of Relativity”
If anybody is interested, I briefly proposed a Big Bang cosmology with massless quantum gravity. My best guess for the elementary particle structure is M-Theory. Here is the preprint (http://philpapers.org/archive/GOESTA-2.pdf).
“If anybody is interested, I briefly proposed a Big Bang cosmology with massless quantum gravity. My best guess for the elementary particle structure is M-Theory. Here is the preprint”
This starts of with:
Semiclassical Theism and the Passage of Planck Times
This paper models God and time in the framework of modern physics. God bridges and simultaneously exists in (1) a universe with infinite tenseless time and (2) a created parallel universe with tensed time and a point origin. The primary attributes of God are inexhaustible love, inexhaustible perception, and inexhaustible force. The model also incorporates modern physics theories that include relativity, the conservation of energy, quantum mechanics, and multiverse geometry. For example, creation out of nothing and divine intervention are subject to physical processes and likewise nomological possibility. I will call this model semiclassical theism.
How about the other way around? Construct Hilbert space as an infinite Cartesian product of tangent spaces of a manifold.
Hi!, Interesting post! I remember reading a similar article from Stephen Wolfram a while back! http://blog.stephenwolfram.com/2015/12/what-is-spacetime-really/
Maybe you should have a chat with him 🙂
Kind of confused about theories not placing an observer in the equations or models of explaining reality. If we add a passive (or active) ‘i’ observer to quantum equations, would there be entropic, gravitational or quantum changes to the quantum equation? Thanks!
Hi Sean.
It would be amazing if you visited the reddit thread for your article and answered some excellent questions raised there.
It would only take few minutes of your time.
https://www.reddit.com/r/Physics/comments/4tgsee/space_emerging_from_quantum_mechanics/
One question raised there is the following :
“So how would this affect the Pauli Exclusion Principle ? Presumably it just becomes a rule about particle entanglement ?”
Thanks.
Has anyone managed to find a link between the growing dark and quantum world and the growth in organic population (humor me).
j.
Dinesh, thanks — I dropped in a couple of short answers, hope that is helpful.
Very interesting. But the idea of space-time being emergent features of QM seems a pretty natural step. As I said a couple of months back, the idea of a graviton (if there is such a thing) being ‘in’ space sounds incoherent on the face of it.
The only problem I have with all of this is that I learned about Hilbert Spaces in maths lectures, not in physics lectures.
As far as I know a Hilbert Space is a generalisation of certain kinds of rules for manipulating generalisations of numbers. In other words, a rule for manipulating symbols, or abstract ideas, not something that exists (unless Max Tegmark is right).
I can’t tell for sure because I understand almost none of the paper, but it looks like the analysis leaves the dimensionality of the emergent spatial manifold entirely unspecified (except that it’s a lot smaller than that of the Hilbert space from which it emerges).
If that’s right, why didn’t you take into account what we think we know about the dimensionality of the space that we actually do observe? Couldn’t this be used to further constrain the Hilbert space quantum state that you start with?
It would be really awesome if out of this model also emerged a limit on the speed of causality.
Ben Goren,
A particle can only interfere with ‘itself’. The double slit particles, or x-rays diffracting from a crystal lattice, or whatever.
Sometimes it seems like this isn’t the case, like when you focus radio waves by interference between multiple broadcasting towers, but if you look at the situation with time running the other way, it is basically the same situation.
If you imagine that the particle between measurements is like a standing wave between all possible non-self interactions ( which occur at various times and locations), then interference and entanglement are the things happening between observed measurements with non-self particles ( but not limited by ‘classical’ pervasive time, only the time-cone of possible interactions.
Moe,
Thanks for the response.
For me, the big unknown, the big mystery, is what is actually happening when one particle interacts with another.
In the double-slit with electrons and a CRT-style phosphor detector, the diffracted electron interacts with a single phosphorus atom in the detector, kicking electrons into different orbits that result in the release of the photon(s?) that we see.
What I don’t understand is the nature of that diffracted-electron-on-phosphorus-atom interaction. What’s actually going on there?
If you had a system with just two particles, rather than the very complex one with a detector with a mole or so of particles, how do those two particles interact? (I imagine a particle collider is much like this, but at insanely high energies.)
Clearly, the complex system must be doing the same thing as the two-particle system, save it’s just one of the many particles in the detector interacting…and the quantum interpretation mystery seems to be a matter of figuring out why that one particle in the detector was the one to interact with the diffracted electron as opposed to some other particle in the detector.
b&
Was thinking about the arrow of time and gravity being linked since there is no negative gravity.
Lord,
The arrow of time is rather well understood in terms of entropy. It simply “falls out” of basic thermodynamics, which itself is really just statistics. The biggest mystery remaining is why the Big Bang had such extraordinarily low entropy…but, once you start with that initial condition, the rest follows. That is, if you have a condition of low entropy, it will create an arrow of time in the direction of increasing entropy.
The entropic arrow of time is one of Sean’s specialties, and you can find a number of great videos (plus a book) linked to on his home page.
Cheers,
b&