I want to tell you about a paper I recently wrote with grad student Grant Remmen, about how much inflation we should expect to have occurred in the early universe. But that paper leans heavily on an earlier one that Grant and I wrote, about phase space and cosmological attractor solutions — one that I never got around to blogging about. So you’re going to hear about that one first! It’s pretty awesome in its own right. (Sadly “cosmological attractors” has nothing at all to do with the hypothetical notion of attractive cosmologists.)
Attractor Solutions in Scalar-Field Cosmology
Grant N. Remmen, Sean M. CarrollModels of cosmological scalar fields often feature “attractor solutions” to which the system evolves for a wide range of initial conditions. There is some tension between this well-known fact and another well-known fact: Liouville’s theorem forbids true attractor behavior in a Hamiltonian system. In universes with vanishing spatial curvature, the field variables (
) specify the system completely, defining an effective phase space. We investigate whether one can define a unique conserved measure on this effective phase space, showing that it exists for m2φ2 potentials and deriving conditions for its existence in more general theories. We show that apparent attractors are places where this conserved measure diverges in the (
This paper investigates a well-known phenomenon in inflationary cosmology: the existence of purported “attractor” solutions. There is a bit of lore that says that an inflationary scalar field might start off doing all sorts of things, but will quickly settle down to a preferred kind of evolution, known as the attractor. But that lore is nominally at odds with a mathematical theorem: in classical mechanics, closed systems never have attractor solutions! That’s because “attractor” means “many initial conditions are driven to the same condition,” while Liouville’s theorem says “a set of initial conditions maintains its volume as it evolves.” So what’s going on?
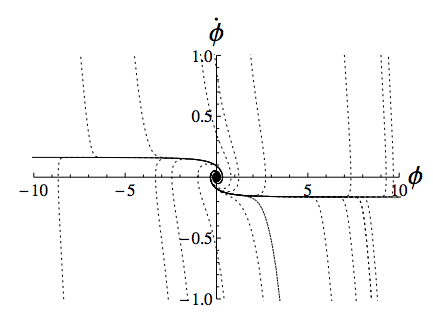
Let’s consider the simplest kind of model: you just have a single scalar field φ, and a potential energy function V(φ), in the context of an expanding universe with no other forms of matter or energy. That fully specifies the model, but then you have to specify the actual trajectory that the field takes as it evolves. Any trajectory is fixed by giving certain initial data in the form of the value of the field φ and its “velocity” 
. For a very simple potential like V(φ) ~ φ2, the trajectories look like this:
This is the “effective phase space” of the model — in a spatially flat universe (and only there), specifying φ and its velocity uniquely determines a trajectory, shown as the lines on the plot. See the dark lines that start horizontally, then spiral toward the origin? Those are the attractor solutions. Other trajectories (dashed lines) basically zoom right to the attractor, then stick nearby for the rest of their evolution. Physically, the expansion of the universe acts as a kind of friction; away from the attractor the friction is too small to matter, but once you get there friction begins to dominate and the the field rolls very slowly. So the idea is that there aren’t really that many different kinds of possible evolution; a “generic” initial condition will just snap onto the attractor and go from there.
This story seems to be in blatant contradiction with Liouville’s Theorem, which roughly says that there cannot be true attractors, because volumes in phase space (the space of initial conditions, i.e. coordinates and momenta) remain constant under time-evolution. Whereas in the picture above, volumes get squeezed to zero because every trajectory flows to the 1-dimensional attractor, and then of course eventually converges to the origin. But we know that the above plot really does show what the trajectories do, and we also know that Liouville’s theorem is correct and does apply to this situation. Our goal for the paper was to show how everything actually fits together.
Obviously (when you think about it, and know a little bit about phase space), the problem is with the coordinates on the above graph. In particular, might be the “velocity” of the field, but it definitely isn’t its “momentum,” in the strict mathematical sense. The canonical momentum is actually 
, where a is the scale factor that measures the size of the universe. And the scale factor changes with time, so there is no simple translation between the nice plot we saw above and the “true” phase space — which should, after all, also include the scale factor itself as well as its canonical momentum.
So there are good reasons of convenience to draw the plot above, but it doesn’t really correspond to phase space. As a result, it looks like there are attractors, although there really aren’t — at least not by the strict mathematical definition. It’s just a convenient, though possibly misleading, nomenclature used by cosmologists.
Still, there is something physically relevant about these cosmological attractors (which we will still call “attractors” even if they don’t match the technical definition). If it’s not “trajectories in phase space focus onto them,” what is it? To investigate this, Grant and I turned to a formalism for defining the measure on the space of trajectories (rather than just points in phase space), originally studied by Gibbons, Hawking, and Stewart and further investigated by Heywood Tam and me a couple of years ago.
The interesting thing about the “GHS measure” on the space of trajectories is that it diverges — becomes infinitely big — for cosmologies that are spatially flat. That is, almost all universes are spatially flat — if you were to pick a homogeneous and isotropic cosmology out of a hat, it would have zero spatial curvature with probability unity. (Which means that the flatness problem you were taught as a young cosmologist is just a sad misunderstanding — more about that later in another post.) That’s fine, but it makes it mathematically tricky to study those flat universes, since the measure is infinity there. Heywood and I proposed a way to regulate this infinity to get a finite answer, but that was a mistake on our part — upon further review, our regularization was not invariant under time-evolution, as it should have been.
That left an open problem — what is the correct measure on the space of flat universes? This is what Grant and I tackled, and basically solved. Long story short, we studied the necessary and sufficient conditions for there to be the right kind of measure on the effective phase space shown in the plot above, and argued that such a measure (1) exists, and (2) is apparently unique, at least in the simple case of a quadratic potential (and probably more generally). That is, we basically reverse-engineered the measure from the requirement that Liouville’s theorem be obeyed!
So there is such a measure, but it’s very different from the naïve “graph-paper measure” that one is tempted to use for the effective phase space plotted above. (A temptation to which almost everyone in the field gives in.) Unsurprisingly, the measure blows up on the attractor, and near the origin. That is, what looks like an attractor when you plot it in these coordinates is really a sign that the density of trajectories grows very large there — which is the least surprising thing in the world, really.
At the end of the day, despite the fact that we mildly scold fellow cosmologists for their sloppy use of the word “attractor,” the physical insights connected to this idea go through essentially unaltered. The field and its velocity are the variables that are most readily observable (or describable) by us, and in terms of these variables the apparent attractor behavior is definitely there. The real usefulness of our paper would come when we wanted to actually use the measure we constructed, for example to calculate the expected amount of inflation in a given model — which is what we did in our more recent paper, to be described later.
This paper, by the way, was one from which I took equations for the blackboards in an episode of Bones. It was fun to hear Richard Schiff, famous as Toby from The West Wing, play a physicist who explains his alibi by saying “I was constructing an invariant measure on the phase space of cosmological spacetimes.”
The episode itself is great, you should watch it if you can. But I warn you — you will cry.
” (Sadly “cosmological attractors” has nothing at all to do with the hypothetical notion of attractive cosmologists.)”
Why “hypothetical”?
A very interesting, but somewhat obscure, branch of classical cosmology is also concerned with attractors, viewing the evolution of the universe as a trajectory in a phase space of the cosmological parameters, etc. Check out a recent review and references therein for more enlightenment.
A brief mention of contrary possibilities can hedge one’s bet. I think Sean has done a fairly good job of this in his explanation. Future observations of the Atacama Array, the James Webb scope when operational, and other scopes could totally contradict today’s cosmology within 10 years from now. Pie’s-in-the-face can be hilarious but not always for those receiving them.
Assumption: Inflation cosmology: a number of different ad hoc hypotheses claimed to be supported by circumstantial evidence.
Assumption: Closed universe: An expanding universe closed by Riemann Geometry, one of the possibilities of General Relativity, which is dependent upon the dark matter hypothesis.
Much of the foundation of any proposal can be no stronger than its initial assumptions/ premises.
I wonder if there are physical cosmological consequences of the mathematical fact that trajectories converge in (phi, phidot) space but not in phase space.
Hi Professor Carroll,
I think this is interesting, I am trying to apply to Caltech very soon and study cosmology with you.
Because of this article I looked up phase space. I now know what a phase space is and by extension attractors. So each position along a trajectory describes the possible state of a system and the trajectory describes its evolution? Am I getting this? I think I am because it’s fascinating. That’s usually a good sign.
That’s exactly right.
Yes, you’re getting it.
Although they don’t use that term, a fascinating study of classical cosmology in the phase-space context is this classic paper by Stabell and Refsdal, which, amazingly, is actually Stabell’s first paper and Refsdal’s first non-gravitational-lens paper (the late Refsdal found fame for essentially single-handedly founding modern gravitational-lens theory).
“Assumption: Closed universe: An expanding universe closed by Riemann Geometry, one of the possibilities of General Relativity, which is dependent upon the dark matter hypothesis. “
Who assumes this? I don’t know anyone, and don’t know of anyone, who assumes this. OK, that’s not a proof, but the burden of proof is on you to point to someone who assumes this. Neither has anyone concluded this from observational data with any significant degree of confidence.
Personally, I like this idea (a spatially closed universe which expands forever, asymptotically approaching exponential expansion) for a variety of reasons. Way back in 1927, Lemaitre wrote of “A homogeneous universe of constant mass and increasing radius accounting for the radial velocity of extra-galactic nebulae” (well, actually he wrote in French, so he wrote of “Un Univers homogène de masse constante et de rayon croissant rendant compte de la vitesse radiale des nébuleuses extra-galactiques”). However, ultimately correctly interpreted observational data will decide this. Now, the jury is out.
The universe is very close to the divide between finite and infinite (at least, if a simple topology is assumed), but we don’t know on which side. Barrow and Shaw (in at least 4 papers; not sure why there are so many such similar papers) predict that Omega+lambd=1.0056 with not much room to move. Maybe even the final analysis of PLANCK data will rule this out. If it is not ruled out, but the allowed range quite small, then chalk one up for Barrow and Shaw.
Wouldn’t a pendulum be an example of an attractor in classical mechanics? It would swing randomly until it settled to one location pointing straight down. Then what would make an attractor different from this type of scenario?
Would it be possible for an attractor to be responsible for the Big Bang event, rather than a quantum fluctuation?
John– A pendulum would only settle down if it had true friction — dissipation. That would not fit into the framework of “Hamiltonian dynamics.” (Because really there are other degrees of freedom that you’re ignoring.)
Sean,
Like John, I would like to know if an attractor could possibly play a role/be responsible a la big bang.
I would have been surprised if he answered that one. I more or less thought of just throwing the idea out there. I never liked the quantum fluctuation explanation, and it has gotten to be a bit of a drab. It seemed like an attractor could be generated by many different unknown causes. If there was some kind of fancy mathematics to show the possibility, then maybe it could link creation to something not even unified in physics, like gravity or something undiscovered in physics altogether.
It would be nice to know we are not here purely by chance. It seems like it would be better if particles inexplicably just derped into barreling into something of which I would really have no idea.
It’s kind of funny, but sometimes I have had the irrational fear of someone actually finding the spark. Hopefully, no one discovers that the spark of the universe wasn’t a mistake in the laws of physics, or maybe it would correct itself in that mistake. I think I would be happy if physics just came closer and closer to that answer without actually knowing exactly what that is.
I always assumed that the Big Bang theory was not only an expansion of the universe but also a dissipation of energy .
“Conservative systems do not have attractors, since the motion is periodic. For dissipative dynamical systems, however, volumes shrink exponentially so attractors have 0 volume in n-dimensional phase space.”
Perhaps I am missing the point. Is the paper showing that the fine tuning problem is irrelevant since all types of initial conditions lead to a flat universe? I may have to go back to the beginning and do the attractor derivations from first principles to make sure that I understand the argument. Is it possible to explain away the horizon problem if the universe is much older instead of using an ad hoc inflation?
Is this something you would feel comfortable commenting on?
Pilot waves
Not sure what the excitement is about. Someone made an analogue system to a theory that has been around forever (http://en.wikipedia.org/wiki/De_Broglie%E2%80%93Bohm_theory), and showed that it does indeed obey the equations that it should. Doesn’t teach us anything new about quantum mechanics.
Pingback: How Much Cosmic Inflation Probably Occurred? | Sean Carroll
Sean, thank you for the awesome articles, your website is always a pleasure to read. I wanted to make a request for a topic that has been nagging me lately and it is related to cosmological attractors. Now, I am fairly knowledgeable about mainstream science but I don’t quite have the chops to bite into some of the more prominent fringe ideas floating around, namely the Electric Universe. All I hear from their crowd is that mainstream science is holding back “the truth” or too afraid to admit it’s wrong, etc. It drives me nuts. They claim that the Electric Universe [hypothesis] debunks the big bang, explains the organization of matter, and easily accounts for things like The Great Attractor.
Personally, I have zero problem with mainstream ideas getting debunked (I love to learn new things about nature and have a rational fear of becoming attached to ideas that could be wrong tomorrow with new observations) and fringe ideas being found to be correct. The attitude of the EU crowd and my lack of ability to address some of their core ideas drives me to ask if you could take a brief look at EU and, if necessary, completely destroy their central claims with your devastating knowledge and verbosity. If you could do this you would be my hero. Some parts of EU are interesting but their smugness demands that they get their asses handled by a professional.
Thanks for your consideration.