I have often talked about the Many-Worlds or Everett approach to quantum mechanics — here’s an explanatory video, an excerpt from From Eternity to Here, and slides from a talk. But I don’t think I’ve ever explained as persuasively as possible why I think it’s the right approach. So that’s what I’m going to try to do here. Although to be honest right off the bat, I’m actually going to tackle a slightly easier problem: explaining why the many-worlds approach is not completely insane, and indeed quite natural. The harder part is explaining why it actually works, which I’ll get to in another post.
Any discussion of Everettian quantum mechanics (“EQM”) comes with the baggage of pre-conceived notions. People have heard of it before, and have instinctive reactions to it, in a way that they don’t have to (for example) effective field theory. Hell, there is even an app, universe splitter, that lets you create new universes from your iPhone. (Seriously.) So we need to start by separating the silly objections to EQM from the serious worries.
The basic silly objection is that EQM postulates too many universes. In quantum mechanics, we can’t deterministically predict the outcomes of measurements. In EQM, that is dealt with by saying that every measurement outcome “happens,” but each in a different “universe” or “world.” Say we think of Schrödinger’s Cat: a sealed box inside of which we have a cat in a quantum superposition of “awake” and “asleep.” (No reason to kill the cat unnecessarily.) Textbook quantum mechanics says that opening the box and observing the cat “collapses the wave function” into one of two possible measurement outcomes, awake or asleep. Everett, by contrast, says that the universe splits in two: in one the cat is awake, and in the other the cat is asleep. Once split, the universes go their own ways, never to interact with each other again.
And to many people, that just seems like too much. Why, this objection goes, would you ever think of inventing a huge — perhaps infinite! — number of different universes, just to describe the simple act of quantum measurement? It might be puzzling, but it’s no reason to lose all anchor to reality.
To see why objections along these lines are wrong-headed, let’s first think about classical mechanics rather than quantum mechanics. And let’s start with one universe: some collection of particles and fields and what have you, in some particular arrangement in space. Classical mechanics describes such a universe as a point in phase space — the collection of all positions and velocities of each particle or field.
What if, for some perverse reason, we wanted to describe two copies of such a universe (perhaps with some tiny difference between them, like an awake cat rather than a sleeping one)? We would have to double the size of phase space — create a mathematical structure that is large enough to describe both universes at once. In classical mechanics, then, it’s quite a bit of work to accommodate extra universes, and you better have a good reason to justify putting in that work. (Inflationary cosmology seems to do it, by implicitly assuming that phase space is already infinitely big.)
That is not what happens in quantum mechanics. The capacity for describing multiple universes is automatically there. We don’t have to add anything.
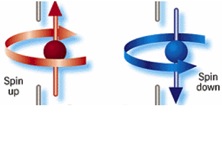The reason why we can state this with such confidence is because of the fundamental reality of quantum mechanics: the existence of superpositions of different possible measurement outcomes. In classical mechanics, we have certain definite possible states, all of which are directly observable. It will be important for what comes later that the system we consider is microscopic, so let’s consider a spinning particle that can have spin-up or spin-down. (It is directly analogous to Schrödinger’s cat: cat=particle, awake=spin-up, asleep=spin-down.) Classically, the possible states are
“spin is up”
or
“spin is down”.
Quantum mechanics says that the state of the particle can be a superposition of both possible measurement outcomes. It’s not that we don’t know whether the spin is up or down; it’s that it’s really in a superposition of both possibilities, at least until we observe it. We can denote such a state like this:
(“spin is up” + “spin is down”).
While classical states are points in phase space, quantum states are “wave functions” that live in something called Hilbert space. Hilbert space is very big — as we will see, it has room for lots of stuff.
To describe measurements, we need to add an observer. It doesn’t need to be a “conscious” observer or anything else that might get Deepak Chopra excited; we just mean a macroscopic measuring apparatus. It could be a living person, but it could just as well be a video camera or even the air in a room. To avoid confusion we’ll just call it the “apparatus.”
In any formulation of quantum mechanics, the apparatus starts in a “ready” state, which is a way of saying “it hasn’t yet looked at the thing it’s going to observe” (i.e., the particle). More specifically, the apparatus is not entangled with the particle; their two states are independent of each other. So the quantum state of the particle+apparatus system starts out like this:
(“spin is up” + “spin is down” ; apparatus says “ready”) (1)
The particle is in a superposition, but the apparatus is not. According to the textbook view, when the apparatus observes the particle, the quantum state collapses onto one of two possibilities:
(“spin is up”; apparatus says “up”)
or
(“spin is down”; apparatus says “down”).
When and how such collapse actually occurs is a bit vague — a huge problem with the textbook approach — but let’s not dig into that right now.
But there is clearly another possibility. If the particle can be in a superposition of two states, then so can the apparatus. So nothing stops us from writing down a state of the form
(spin is up ; apparatus says “up”)
+ (spin is down ; apparatus says “down”). (2)
The plus sign here is crucial. This is not a state representing one alternative or the other, as in the textbook view; it’s a superposition of both possibilities. In this kind of state, the spin of the particle is entangled with the readout of the apparatus.
What would it be like to live in a world with the kind of quantum state we have written in (2)? It might seem a bit unrealistic at first glance; after all, when we observe real-world quantum systems it always feels like we see one outcome or the other. We never think that we ourselves are in a superposition of having achieved different measurement outcomes.
This is where the magic of decoherence comes in. (Everett himself actually had a clever argument that didn’t use decoherence explicitly, but we’ll take a more modern view.) I won’t go into the details here, but the basic idea isn’t too difficult. There are more things in the universe than our particle and the measuring apparatus; there is the rest of the Earth, and for that matter everything in outer space. That stuff — group it all together and call it the “environment” — has a quantum state also. We expect the apparatus to quickly become entangled with the environment, if only because photons and air molecules in the environment will keep bumping into the apparatus. As a result, even though a state of this form is in a superposition, the two different pieces (one with the particle spin-up, one with the particle spin-down) will never be able to interfere with each other. Interference (different parts of the wave function canceling each other out) demands a precise alignment of the quantum states, and once we lose information into the environment that becomes impossible. That’s decoherence.
Once our quantum superposition involves macroscopic systems with many degrees of freedom that become entangled with an even-larger environment, the different terms in that superposition proceed to evolve completely independently of each other. It is as if they have become distinct worlds — because they have. We wouldn’t think of our pre-measurement state (1) as describing two different worlds; it’s just one world, in which the particle is in a superposition. But (2) has two worlds in it. The difference is that we can imagine undoing the superposition in (1) by carefully manipulating the particle, but in (2) the difference between the two branches has diffused into the environment and is lost there forever.
All of this exposition is building up to the following point: in order to describe a quantum state that includes two non-interacting “worlds” as in (2), we didn’t have to add anything at all to our description of the universe, unlike the classical case. All of the ingredients were already there!
Our only assumption was that the apparatus obeys the rules of quantum mechanics just as much as the particle does, which seems to be an extremely mild assumption if we think quantum mechanics is the correct theory of reality. Given that, we know that the particle can be in “spin-up” or “spin-down” states, and we also know that the apparatus can be in “ready” or “measured spin-up” or “measured spin-down” states. And if that’s true, the quantum state has the built-in ability to describe superpositions of non-interacting worlds. Not only did we not need to add anything to make it possible, we had no choice in the matter. The potential for multiple worlds is always there in the quantum state, whether you like it or not.
The next question would be, do multiple-world superpositions of the form written in (2) ever actually come into being? And the answer again is: yes, automatically, without any additional assumptions. It’s just the ordinary evolution of a quantum system according to Schrödinger’s equation. Indeed, the fact that a state that looks like (1) evolves into a state that looks like (2) under Schrödinger’s equation is what we mean when we say “this apparatus measures whether the spin is up or down.”
The conclusion, therefore, is that multiple worlds automatically occur in quantum mechanics. They are an inevitable part of the formalism. The only remaining question is: what are you going to do about it? There are three popular strategies on the market: anger, denial, and acceptance.
The “anger” strategy says “I hate the idea of multiple worlds with such a white-hot passion that I will change the rules of quantum mechanics in order to avoid them.” And people do this! In the four options listed here, both dynamical-collapse theories and hidden-variable theories are straightforward alterations of the conventional picture of quantum mechanics. In dynamical collapse, we change the evolution equation, by adding some explicitly stochastic probability of collapse. In hidden variables, we keep the Schrödinger equation intact, but add new variables — hidden ones, which we know must be explicitly non-local. Of course there is currently zero empirical evidence for these rather ad hoc modifications of the formalism, but hey, you never know.
The “denial” strategy says “The idea of multiple worlds is so profoundly upsetting to me that I will deny the existence of reality in order to escape having to think about it.” Advocates of this approach don’t actually put it that way, but I’m being polemical rather than conciliatory in this particular post. And I don’t think it’s an unfair characterization. This is the quantum Bayesianism approach, or more generally “psi-epistemic” approaches. The idea is to simply deny that the quantum state represents anything about reality; it is merely a way of keeping track of the probability of future measurement outcomes. Is the particle spin-up, or spin-down, or both? Neither! There is no particle, there is no spoon, nor is there the state of the particle’s spin; there is only the probability of seeing the spin in different conditions once one performs a measurement. I advocate listening to David Albert’s take at our WSF panel.
The final strategy is acceptance. That is the Everettian approach. The formalism of quantum mechanics, in this view, consists of quantum states as described above and nothing more, which evolve according to the usual Schrödinger equation and nothing more. The formalism predicts that there are many worlds, so we choose to accept that. This means that the part of reality we experience is an indescribably thin slice of the entire picture, but so be it. Our job as scientists is to formulate the best possible description of the world as it is, not to force the world to bend to our pre-conceptions.
Such brave declarations aren’t enough on their own, of course. The fierce austerity of EQM is attractive, but we still need to verify that its predictions map on to our empirical data. This raises questions that live squarely at the physics/philosophy boundary. Why does the quantum state branch into certain kinds of worlds (e.g., ones where cats are awake or ones where cats are asleep) and not others (where cats are in superpositions of both)? Why are the probabilities that we actually observe given by the Born Rule, which states that the probability equals the wave function squared? In what sense are there probabilities at all, if the theory is completely deterministic?
These are the serious issues for EQM, as opposed to the silly one that “there are just too many universes!” The “why those states?” problem has essentially been solved by the notion of pointer states — quantum states split along lines that are macroscopically robust, which are ultimately delineated by the actual laws of physics (the particles/fields/interactions of the real world). The probability question is trickier, but also (I think) solvable. Decision theory is one attractive approach, and Chip Sebens and I are advocating self-locating uncertainty as a friendly alternative. That’s the subject of a paper we just wrote, which I plan to talk about in a separate post.
There are other silly objections to EQM, of course. The most popular is probably the complaint that it’s not falsifiable. That truly makes no sense. It’s trivial to falsify EQM — just do an experiment that violates the Schrödinger equation or the principle of superposition, which are the only things the theory assumes. Witness a dynamical collapse, or find a hidden variable. Of course we don’t see the other worlds directly, but — in case we haven’t yet driven home the point loudly enough — those other worlds are not added on to the theory. They come out automatically if you believe in quantum mechanics. If you have a physically distinguishable alternative, by all means suggest it — the experimenters would love to hear about it. (And true alternatives, like GRW and Bohmian mechanics, are indeed experimentally distinguishable.)
Sadly, most people who object to EQM do so for the silly reasons, not for the serious ones. But even given the real challenges of the preferred-basis issue and the probability issue, I think EQM is way ahead of any proposed alternative. It takes at face value the minimal conceptual apparatus necessary to account for the world we see, and by doing so it fits all the data we have ever collected. What more do you want from a theory than that?
Neil Bates: re your question, the brief answer IMHO is that wavefunction is real and that detection involves something akin to the optical Fourier transform. You’re using one extended entity, an electron, to detect another, the photon. Detection at one slit transforms to photon to a pointlike entity at the slit so it goes through one slit only. Everything else builds on top of that.
Briefly, re strong correlations: Bell and others worked through the math showing that local properties just can’t produces the kind of results that we get. That is math and you can find the argument all over, you don’t need me for that. It’s your job to show that a long-standing and in-good-standing orthodox proof is “wrong” after all these years. Sure, I shouldn’t be dogmatic but I am not convinced by alternative claims any more than by alternative claims about relativity or there being an aether or whatnot. As for credentials, I merely noted that I am *not* an expert but try to stay abreast of what the theory is saying.
Re: Bell’s theorem, people who think that Bell only showed that hidden variable theories have to be non-local, should try actually reading Bell. He’s quite clear about this, especially in his later papers where he is specifically addressing this long-standing misconception. “La Nouvelle Cuisine” (reprinted in the 2nd edition of Speakable and Unspeakable) is particularly accessible and clear. A careful systematic review can be found here:
http://www.scholarpedia.org/article/Bell%27s_theorem
Travis, such an analysis misses the main thrust of why locality alone reaches the contradiction. The big problem is that the assumption that the orientation of the detectors does not affect the measurement is not warranted because values depend on the set of commuting operators in which the system is characterized. This is the contextuality of the Kochen-Specker theorem. In most frameworks, locality implies noncontextuality and you get Bell’s Theorem. However, there are entire sets of interpretations of quantum mechanics that avoid the noncontextuality of the KS theorem and its connection to locality, like some modal interpretations: http://plato.stanford.edu/entries/qm-modal/
Daniel, I certainly do not agree that modal interpretations provide counter-examples to Bell’s claim that nonlocality is required for empirical adequacy, if that’s what you meant to suggest. (Of course, one can explain the correlations locally by violating the “no conspiracies” assumption, but that’s very hard to take seriously.) But this isn’t really the place to discuss that.
I still want to understand better what the ontology of Everettian QM is, according to our host.
PS, Daniel, I just read through some earlier comments including yours about the red/blue ball. You really need to read Bell’s papers (e.g., “La Nouvelle Cuisine”). It’s clear that you don’t understand what he claimed and on what grounds. You present — as if it refutes his conclusions — something that is a part of his ground-clearing setup.
Shodan this forum supports LaTex, please provide a detailed proof as to why we need an i for unitary evolution because if you cannot derive it then you do not understand it. This forum exemplifies much of the drama in academia. Instead of helping people to make logical sense of the theory it quickly turns into a pissing contest of who is smarter. No one seems to love this subject instead everyone wants to pretend that they know what they are doing. Shodan we cannot teach if you don’t think that you have anything to learn. I don’t listen to what Sean Carroll says because I believe he is a great quantum physicist. I am more interested in how he thinks about general relativity and cosmology.
Travis, I mean only to point out that interpretations exist that conserve a notion of locality and are compatible with QM. Whether you take them seriously or not is immaterial provided their consistency. I don’t personally subscribe to them either, the interpretation I subscribe to would conclude that quantum is nonlocal from bell’s theorem. I accept this is not the only interpretation. If you have an explicit objection, I’m really willing to read it and discuss it. Telling me I don’t understand and providing no further clarification beyond that doesn’t really help either of us out.
The red/blue ball example was not meant to be literally analogous, but rather a toy example to illustrate the role of “distance” in the measurement. Like most analogies though, it seems it’s taken away from my point! What you’re calling “non-locality” would hold irrespective of a Poincare or Galilei geometry. This “non-locality” can be derived whether there’s a speed of light or not. It can be derived even in the absence of an embedding geometry for these particles.
This “contradiction” is derived solely in SU(2) (which is the spin group of the Galilei group), which has no concept of locality that can be violated. It holds for any non-commutative Lie algebra though, we just focus on the spin groups.
Now that I think of it, I actually have never seen a derivation of Bell’s Theorem in SL(2, C) which is the spin group of the Poincare group and thus the correct group to carry out this analysis. Since it contains SU(2)xSU(2) as a subgroup, and regardless is a rotation/spin group, I would think it would hold given the non-commutable basis for rotation operators, but there might be some differences. Do you know of any references that discuss this?
Anyway, since this result is a general feature of the non-commutative algebra of quantum mechanics completely tangential to embedding geometry, it is not hard to imagine that there are interpretations that conserve a sense of locality while still being consistent with this result.
‘The pilot-wave dynamics of walking droplets’
https://www.youtube.com/watch?v=nmC0ygr08tE
What waves in a double slit experiment is the dark matter.
‘What If There’s a Way to Explain Quantum Physics Without the Probabilistic Weirdness?’
http://www.smithsonianmag.com/smart-news/what-if-theres-way-explain-quantum-physics-without-all-probabilistic-weirdness-180951914/#JEoZGUo23dbMGJly.16
“Known as “pilot wave theory” this line of thinking goes that, rather than electrons and other things being both quasi-particles and quasi-waves, the electron is a discrete particle that is being carried along by a separate wave. What this wave is made of no one knows.”
‘Redefining Dark Matter – Wave Instead Of Particle’
http://www.science20.com/news_articles/redefining_dark_matter_wave_instead_of_particle-139771
“Tom Broadhurst, an Ikerbasque researcher at the University of the Basque Country (UPV/EHU), explains that, “guided by the initial simulations of the formation of galaxies in this context, we have reinterpreted cold dark matter as a Bose-Einstein condensate”. So, “the ultra-light bosons forming the condensate share the same quantum wave function, so disturbance patterns are formed on astronomic scales in the form of large-scale waves”.”
“This opens up the possibility that dark matter could be regarded as a very cold quantum fluid”
Travis,
Actually, it is clear that you are the one who does not understand quantum mechanics. As has been explained before, you are insisting non-locality is essential because you are assuming classical degrees of freedom a priori and then attempting to construct an explanation for strong correlations in the context of these degrees of freedom. Instead, you have to understand that in quantum mechanics, unlike classical mechanics, observables have a very different logical structure. They don’t commute. This is a fundamental feature of QM and does not need to be derived from some classical framework. In fact, the opposite is the case. Classical degrees of freedom are an approximation, a limit of the rules of QM. In no way, shape or form, does the orientation of a detector effect the results measured by the second detector.
Once again, I point you to the very clear explanation of such correlations: Section 7 of this paper: http://arxiv.org/pdf/1308.5290v2.pdf
Shodan brings up a good point. Bell’s theorem can be derived as a consequence of the KS-theorem, and one of the necessary assumptions of KS theory is that a value function can be defined on operators in a linear manner. This assumption, whether stated or not, is a kind of hidden variable hypothesis. This is equivalent to arguing in the Bell theorem, that a joint probability distribution can be classically defined as if the two experiments are independent. Clearly the non-commutativity of the operators involved do not allow a reduction to a classical valued representation of their action.
The infinite dimensional Hilbert space is splitting into two separate universes I don’t have long please help me!
Shodan, I read sections 7 and 8 of your (?) paper. There is no explanation of the correlations there, just some confused and misleading talking points that in no way confront Bell’s actual argument. Read Bell.
Like many others I still can’t get my head around the concept of the ‘observer’ as used here. Surely a ‘macroscopic’ object is simply a collection of microscopic objects so I can see no clear distinction between the ‘apparatus’ (as the term is used here) and what it is observing.
How ‘big’ does ‘macroscopic’ have to be.
If the apparatus can be a video camera, then presumably it can be some molecular machine, in principle.
So could the apparatus be smaller than the superposition it is measuring?
For example could the superposition be something like a buckyball and being measured by an apparatus that is a molecular machine that is smaller than a buckyball?
Robin,
In terms of de Broglie wave mechanics and double solution theory ‘observation’ is another term for detection.
In de Broglie wave mechanics and double solution theory the particle is guided by an associated physical wave.
The stronger the particle is detected the more it loses its cohesion with its associated wave, the less it is guided by its associated wave, the more it continues on the trajectory it was traveling.
Bell’s theorem is, of course, a rigorous deductive proof of his conclusion, given his premises. And his premise makes perfect sense in a local realist theory. A lot of advocates of hidden variables, and various anti-quantum crackpots may insist that Bell’s theorem casts a much wider net than this, but they are wrong. Bell himself was wrong on this, to be sure.
Most importantly, the notion of locality (or local causality) that Bell defines only makes sense in the first place if you assume that reality consists in some way of ordinary, commuting numbers that are localized to spacetime points. (e.g. the lambda in his definition of locality.) They may be unknown numbers, characterized by some probability distribution (hence the integral over lambda in Bell’s definition of locality), but they must be present. However, quantum mechanics repudiates this claim about reality in the first place, so Bell’s definition of locality makes no sense as a definition within QM itself.
There is, to be sure, a standard definition of locality in quantum mechanics. Operators rather than numbers are localized to spacetime points, so locality is defined by operators at spacelike separation commuting. This definition makes sense because causal, dynamical laws are encoded via commutation relations; e.g. [A,H] = idA/dt. And relativistic quantum field theory does in fact obey this; it is perfectly local. (It is moreover covariant under Lorentz transformations, which is really *the* hurdle a theory needs to jump to satisfy special relativity.)
Quantum mechanics is Bell-nonlocal, to be sure; it fails to satisfy Bell’s notion of locality. But Bell’s notion makes no sense for QM, so this really doesn’t matter for either QM to be conceptually sensible, nor for it to be compatible with special relativity. However, Bell’s notion of locality makes perfect sense for a theory of hidden variables, so the failure of any such theory to be Bell-local that reproduces QM’s predictions is actually an indictment against the hidden variable theory. This is why physicists say Bell’s theorem rules out local hidden variable theories, despite what Bell’s opinion on the matter is.
Whatthestuff, could you say more about “double solution theory”, and is that a particular species similar to dB-B, or is it a name for the general category (particle and wave both present) of such theories? Note that everyone still debates all this, it does not have a clear consensus or even clear background framing to base from.
Shintaro: Sure, the meaningfulness of BT depends on the beables and what kind of background framing you have, etc. I think of it as a conditional proof. *If* you want to recover a “realist” QM and do imagine local properties in order to explain the strong correlations, then you will fail, etc. Sure you can just blow it all off and say it’s mysterious and not have to represent anything anyway, etc. The BT is a conditional proof of limitations.
Re: Alanl @
June 30, 2014 at 7:41 pm
According to my layman’s understanding of Dr. Carroll’s post, the “splitting” of different worlds takes place such that there is consistency in each of the splits. There cannot be a single, un-superimposed world in which observer A sees the cat dead and observer B sees the cat as alive. Why? because everything in each split-world is entangled (in a QM sense), or can be entangled, and there is no way to entangle (i.e., exchange information) between two inconsistent states. Your thought experiment is basically the same as Bell’s experiment (in which two entangled particles always have the same property no matter the distance between their measurements or which particle is measured first).
Re: conservation laws.
I cannot see how there can be any problem with conservation laws, as these can only be measured in each entangled world/universe, such as the one in which we all find ourselves having this discussion. In other words, if the multiverse had a conservation law so that energy was divided between universes at the time of a split, we would never have observed conservation of energy in our mutual (version of the) universe and we would not have formulated the law to begin with. (Some of you might be then arguing that the version of MWI which applied to such a multiverse was wrong because it violated non-conservation of energy.)
Niels Bates,
de Broglie-Bohm theory is incorrectly named as de Broglie disagreed with it. de Broglie-Bohm theory should be referred to as Bohmian mechanics. In Bohmian mechanics the wave function is considered to be physically real. In de Broglie wave mechanics and double solution theory there is the physical wave which guides the particle and the wave function which is a mathematical construct only and is used to determine the probabilistic results of experiments.
Everyone has a bias
Shintaro, You say that Bell’s notion of locality “makes no sense for QM”. I don’t even really know what you think you mean by that: surely Bell’s formulation “makes sense” (independent of any particular candidate theory) and it is easy to see that QM violates it. But let’s leave that aside. The fundamental point here is that, as Bell pointed out very clearly, the very issue of “locality” is about what a theory says is going on physically: “It is in terms of local beables that we can hope to formulate some notion of local causality.” A theory that says *nothing* about what’s going on physically (but is instead, say, exclusively about what “observers” will “experience”) is outside the domain of the locality/nonlocality issue. Similarly, a theory whose ontology is at best vague and muddled is not ready for prime time: you simply can’t tell whether a theory says that physical goings-on respect relativity’s supposed prohibition on superluminal causation, until it is crystal clear what a theory says the physical goings-on *are*.
If you want to claim that (ordinary) QM is local, you thus need to say clearly and precisely what it says is going on physically, i.e., what the local beables of the theory are, what the ontology is. So lay it out. Tell us what ordinary QM says exists, exactly, and what laws govern the existents’ behavior. Then (and only then) will it be possible to judge whether the theory is local or nonlocal.
I strongly suspect that you’ll balk, claiming that the very idea of specifying the ontology clearly is equivalent to endorsing hidden variables or is in some other way counter to the proper quantum spirit. In that event, we will just agree to disagree. But anybody should be able to see that as long as you refuse to say clearly what your theory says is actually happening physically in the world, your claim that the theory is “local” is, at best, hot air.
Everyone might have a bias, however, there are more and less correct explanations as to what occurs physically in nature. There is also the desire to relate general relativity and quantum mechanics.
‘Redefining Dark Matter – Wave Instead Of Particle’
http://www.science20.com/news_articles/redefining_dark_matter_wave_instead_of_particle-139771
“Tom Broadhurst, an Ikerbasque researcher at the University of the Basque Country (UPV/EHU), explains that, “guided by the initial simulations of the formation of galaxies in this context, we have reinterpreted cold dark matter as a Bose-Einstein condensate”. So, “the ultra-light bosons forming the condensate share the same quantum wave function, so disturbance patterns are formed on astronomic scales in the form of large-scale waves”.”
“This opens up the possibility that dark matter could be regarded as a very cold quantum fluid”
In de Broglie wave mechanics and double solution theory what waves is the very cold quantum dark matter fluid.
What ripples when galaxy clusters collide is what waves in a double slit experiment; the dark matter.
Einstein’s gravitational wave is de Broglie’s wave of wave-particle duality; both are waves in the dark matter.
Dark matter displaced by the particles of matter which exist in it and move through it relates general relativity and quantum mechanics.
I know the debate is the interpretation of the wavefunction, but this is a smart group who might be able to guide me on a different problem. The redshift and acceleration of galaxies away from each other is evidence of a force. However, of the four fundamental forces only two of them, the gravitational and electromagnetic force, have a range large enough to perturb galaxies. The electromagnetic force is the only force that we know of that has an infinite range and is also repulsive in nature. Have we done enough work to falsify an electromagnetic explanation? I expect down votes for this post because of course it is an inflationary dark energy from that explosion of space time and matter. But just for fun what if it is the electromagnetic force? How would you set up the problem?
@Random dx/dt tangent: The problem with using electromagnetism for repulsive force between galaxies is that the matter as a whole is electrically neutral , otherwise we would have been dead long time ago by electrical shocks! All stable atoms and electromagnetic waves are neutral. The positive and negative charges have to be separated before any electrical device works.
Travis. I can certainly agree to disagree with someone who thinks that quantum mechanics, which has been successfully describing atomic, nuclear, particle, solid state, and condensed matter physics for nearly a hundred years is, “not ready for prime time.” Whether you like it or not, there is a notion of locality in quantum physics that does not require any assumptions about local beables (or “ontology” or “metaphysics” or other such nonsense) at all, and it works splendidly. Physics has no need to be held back by outdated pictures of reality, and certainly the word “physically” does not have to mean what Bell wants it to mean. (The way you use the word “physically,” just as the way you and Bell use the word “local,” is deliberately aimed to contradict quantum mechanics. Essentially, you’re begging the question in your dismissal of QM, so your argument is completely vacuous.)