I have often talked about the Many-Worlds or Everett approach to quantum mechanics — here’s an explanatory video, an excerpt from From Eternity to Here, and slides from a talk. But I don’t think I’ve ever explained as persuasively as possible why I think it’s the right approach. So that’s what I’m going to try to do here. Although to be honest right off the bat, I’m actually going to tackle a slightly easier problem: explaining why the many-worlds approach is not completely insane, and indeed quite natural. The harder part is explaining why it actually works, which I’ll get to in another post.
Any discussion of Everettian quantum mechanics (“EQM”) comes with the baggage of pre-conceived notions. People have heard of it before, and have instinctive reactions to it, in a way that they don’t have to (for example) effective field theory. Hell, there is even an app, universe splitter, that lets you create new universes from your iPhone. (Seriously.) So we need to start by separating the silly objections to EQM from the serious worries.
The basic silly objection is that EQM postulates too many universes. In quantum mechanics, we can’t deterministically predict the outcomes of measurements. In EQM, that is dealt with by saying that every measurement outcome “happens,” but each in a different “universe” or “world.” Say we think of Schrödinger’s Cat: a sealed box inside of which we have a cat in a quantum superposition of “awake” and “asleep.” (No reason to kill the cat unnecessarily.) Textbook quantum mechanics says that opening the box and observing the cat “collapses the wave function” into one of two possible measurement outcomes, awake or asleep. Everett, by contrast, says that the universe splits in two: in one the cat is awake, and in the other the cat is asleep. Once split, the universes go their own ways, never to interact with each other again.
And to many people, that just seems like too much. Why, this objection goes, would you ever think of inventing a huge — perhaps infinite! — number of different universes, just to describe the simple act of quantum measurement? It might be puzzling, but it’s no reason to lose all anchor to reality.
To see why objections along these lines are wrong-headed, let’s first think about classical mechanics rather than quantum mechanics. And let’s start with one universe: some collection of particles and fields and what have you, in some particular arrangement in space. Classical mechanics describes such a universe as a point in phase space — the collection of all positions and velocities of each particle or field.
What if, for some perverse reason, we wanted to describe two copies of such a universe (perhaps with some tiny difference between them, like an awake cat rather than a sleeping one)? We would have to double the size of phase space — create a mathematical structure that is large enough to describe both universes at once. In classical mechanics, then, it’s quite a bit of work to accommodate extra universes, and you better have a good reason to justify putting in that work. (Inflationary cosmology seems to do it, by implicitly assuming that phase space is already infinitely big.)
That is not what happens in quantum mechanics. The capacity for describing multiple universes is automatically there. We don’t have to add anything.
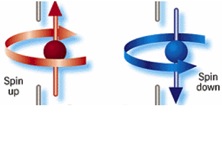The reason why we can state this with such confidence is because of the fundamental reality of quantum mechanics: the existence of superpositions of different possible measurement outcomes. In classical mechanics, we have certain definite possible states, all of which are directly observable. It will be important for what comes later that the system we consider is microscopic, so let’s consider a spinning particle that can have spin-up or spin-down. (It is directly analogous to Schrödinger’s cat: cat=particle, awake=spin-up, asleep=spin-down.) Classically, the possible states are
“spin is up”
or
“spin is down”.
Quantum mechanics says that the state of the particle can be a superposition of both possible measurement outcomes. It’s not that we don’t know whether the spin is up or down; it’s that it’s really in a superposition of both possibilities, at least until we observe it. We can denote such a state like this:
(“spin is up” + “spin is down”).
While classical states are points in phase space, quantum states are “wave functions” that live in something called Hilbert space. Hilbert space is very big — as we will see, it has room for lots of stuff.
To describe measurements, we need to add an observer. It doesn’t need to be a “conscious” observer or anything else that might get Deepak Chopra excited; we just mean a macroscopic measuring apparatus. It could be a living person, but it could just as well be a video camera or even the air in a room. To avoid confusion we’ll just call it the “apparatus.”
In any formulation of quantum mechanics, the apparatus starts in a “ready” state, which is a way of saying “it hasn’t yet looked at the thing it’s going to observe” (i.e., the particle). More specifically, the apparatus is not entangled with the particle; their two states are independent of each other. So the quantum state of the particle+apparatus system starts out like this:
(“spin is up” + “spin is down” ; apparatus says “ready”) (1)
The particle is in a superposition, but the apparatus is not. According to the textbook view, when the apparatus observes the particle, the quantum state collapses onto one of two possibilities:
(“spin is up”; apparatus says “up”)
or
(“spin is down”; apparatus says “down”).
When and how such collapse actually occurs is a bit vague — a huge problem with the textbook approach — but let’s not dig into that right now.
But there is clearly another possibility. If the particle can be in a superposition of two states, then so can the apparatus. So nothing stops us from writing down a state of the form
(spin is up ; apparatus says “up”)
+ (spin is down ; apparatus says “down”). (2)
The plus sign here is crucial. This is not a state representing one alternative or the other, as in the textbook view; it’s a superposition of both possibilities. In this kind of state, the spin of the particle is entangled with the readout of the apparatus.
What would it be like to live in a world with the kind of quantum state we have written in (2)? It might seem a bit unrealistic at first glance; after all, when we observe real-world quantum systems it always feels like we see one outcome or the other. We never think that we ourselves are in a superposition of having achieved different measurement outcomes.
This is where the magic of decoherence comes in. (Everett himself actually had a clever argument that didn’t use decoherence explicitly, but we’ll take a more modern view.) I won’t go into the details here, but the basic idea isn’t too difficult. There are more things in the universe than our particle and the measuring apparatus; there is the rest of the Earth, and for that matter everything in outer space. That stuff — group it all together and call it the “environment” — has a quantum state also. We expect the apparatus to quickly become entangled with the environment, if only because photons and air molecules in the environment will keep bumping into the apparatus. As a result, even though a state of this form is in a superposition, the two different pieces (one with the particle spin-up, one with the particle spin-down) will never be able to interfere with each other. Interference (different parts of the wave function canceling each other out) demands a precise alignment of the quantum states, and once we lose information into the environment that becomes impossible. That’s decoherence.
Once our quantum superposition involves macroscopic systems with many degrees of freedom that become entangled with an even-larger environment, the different terms in that superposition proceed to evolve completely independently of each other. It is as if they have become distinct worlds — because they have. We wouldn’t think of our pre-measurement state (1) as describing two different worlds; it’s just one world, in which the particle is in a superposition. But (2) has two worlds in it. The difference is that we can imagine undoing the superposition in (1) by carefully manipulating the particle, but in (2) the difference between the two branches has diffused into the environment and is lost there forever.
All of this exposition is building up to the following point: in order to describe a quantum state that includes two non-interacting “worlds” as in (2), we didn’t have to add anything at all to our description of the universe, unlike the classical case. All of the ingredients were already there!
Our only assumption was that the apparatus obeys the rules of quantum mechanics just as much as the particle does, which seems to be an extremely mild assumption if we think quantum mechanics is the correct theory of reality. Given that, we know that the particle can be in “spin-up” or “spin-down” states, and we also know that the apparatus can be in “ready” or “measured spin-up” or “measured spin-down” states. And if that’s true, the quantum state has the built-in ability to describe superpositions of non-interacting worlds. Not only did we not need to add anything to make it possible, we had no choice in the matter. The potential for multiple worlds is always there in the quantum state, whether you like it or not.
The next question would be, do multiple-world superpositions of the form written in (2) ever actually come into being? And the answer again is: yes, automatically, without any additional assumptions. It’s just the ordinary evolution of a quantum system according to Schrödinger’s equation. Indeed, the fact that a state that looks like (1) evolves into a state that looks like (2) under Schrödinger’s equation is what we mean when we say “this apparatus measures whether the spin is up or down.”
The conclusion, therefore, is that multiple worlds automatically occur in quantum mechanics. They are an inevitable part of the formalism. The only remaining question is: what are you going to do about it? There are three popular strategies on the market: anger, denial, and acceptance.
The “anger” strategy says “I hate the idea of multiple worlds with such a white-hot passion that I will change the rules of quantum mechanics in order to avoid them.” And people do this! In the four options listed here, both dynamical-collapse theories and hidden-variable theories are straightforward alterations of the conventional picture of quantum mechanics. In dynamical collapse, we change the evolution equation, by adding some explicitly stochastic probability of collapse. In hidden variables, we keep the Schrödinger equation intact, but add new variables — hidden ones, which we know must be explicitly non-local. Of course there is currently zero empirical evidence for these rather ad hoc modifications of the formalism, but hey, you never know.
The “denial” strategy says “The idea of multiple worlds is so profoundly upsetting to me that I will deny the existence of reality in order to escape having to think about it.” Advocates of this approach don’t actually put it that way, but I’m being polemical rather than conciliatory in this particular post. And I don’t think it’s an unfair characterization. This is the quantum Bayesianism approach, or more generally “psi-epistemic” approaches. The idea is to simply deny that the quantum state represents anything about reality; it is merely a way of keeping track of the probability of future measurement outcomes. Is the particle spin-up, or spin-down, or both? Neither! There is no particle, there is no spoon, nor is there the state of the particle’s spin; there is only the probability of seeing the spin in different conditions once one performs a measurement. I advocate listening to David Albert’s take at our WSF panel.
The final strategy is acceptance. That is the Everettian approach. The formalism of quantum mechanics, in this view, consists of quantum states as described above and nothing more, which evolve according to the usual Schrödinger equation and nothing more. The formalism predicts that there are many worlds, so we choose to accept that. This means that the part of reality we experience is an indescribably thin slice of the entire picture, but so be it. Our job as scientists is to formulate the best possible description of the world as it is, not to force the world to bend to our pre-conceptions.
Such brave declarations aren’t enough on their own, of course. The fierce austerity of EQM is attractive, but we still need to verify that its predictions map on to our empirical data. This raises questions that live squarely at the physics/philosophy boundary. Why does the quantum state branch into certain kinds of worlds (e.g., ones where cats are awake or ones where cats are asleep) and not others (where cats are in superpositions of both)? Why are the probabilities that we actually observe given by the Born Rule, which states that the probability equals the wave function squared? In what sense are there probabilities at all, if the theory is completely deterministic?
These are the serious issues for EQM, as opposed to the silly one that “there are just too many universes!” The “why those states?” problem has essentially been solved by the notion of pointer states — quantum states split along lines that are macroscopically robust, which are ultimately delineated by the actual laws of physics (the particles/fields/interactions of the real world). The probability question is trickier, but also (I think) solvable. Decision theory is one attractive approach, and Chip Sebens and I are advocating self-locating uncertainty as a friendly alternative. That’s the subject of a paper we just wrote, which I plan to talk about in a separate post.
There are other silly objections to EQM, of course. The most popular is probably the complaint that it’s not falsifiable. That truly makes no sense. It’s trivial to falsify EQM — just do an experiment that violates the Schrödinger equation or the principle of superposition, which are the only things the theory assumes. Witness a dynamical collapse, or find a hidden variable. Of course we don’t see the other worlds directly, but — in case we haven’t yet driven home the point loudly enough — those other worlds are not added on to the theory. They come out automatically if you believe in quantum mechanics. If you have a physically distinguishable alternative, by all means suggest it — the experimenters would love to hear about it. (And true alternatives, like GRW and Bohmian mechanics, are indeed experimentally distinguishable.)
Sadly, most people who object to EQM do so for the silly reasons, not for the serious ones. But even given the real challenges of the preferred-basis issue and the probability issue, I think EQM is way ahead of any proposed alternative. It takes at face value the minimal conceptual apparatus necessary to account for the world we see, and by doing so it fits all the data we have ever collected. What more do you want from a theory than that?
Shodan says:
June 30, 2014 at 10:51 pm
Bob,
I would instead say the logic compels us to accept either-or. If we reject a realist interpretation of QM, then any obligation to suppose non-local interactions leaves with it.
___________________
Well that’s the crux of it, this is my problem with instrumentalism. While I acknowledge the great value of the mathematical formalism developed in the Consistent ( Decoherent) Histories interpretation, I think non realism is a bad philosophy.
Sean Carroll, you sold me on EQM. I like the decision theory approach; it gives me flashbacks to Fermat’s Principle.
“(spin is up ; apparatus says “up”)
+ (spin is down ; apparatus says “down”). (2)
The plus sign here is crucial. This is not a state representing one alternative or the other, as in the textbook view; it’s a superposition of both possibilities. In this kind of state, the spin of the particle is entangled with the readout of the apparatus.”
I’m not sure I follow here. By “entangled” do you mean that the up or down indication on the apparatus is what determines or partially determines the spin?
From an ongoing discussion
My personal view is that there is a dynamical process when the measurement occurs, that results in a particular outcome. It might involve a change in the SWE and/or non reversibility. But I also wonder whether such a process, if it could be defined, would make QM deterministic and/or be a violation of the prohibition of a local hidden variable. So it is possible that no such dynamic description exists. However, my ignorance of the measurement process does not restrict me from demonstrating holes in woo woo theories such a MW, or to insist that there is an obvious contradiction between countably infinite Rydberg states and the HP..
—————————————————————-
You do realize dynamic collapse theories involve an empirical and not an interpretation question. If you don’t understand this you’re hardly alone , this seems to be a common confusion. Were there empirical evidence of a collapse, we wouldn’t be arguing any more. With regard to your woo-woo comment, there are only two grounds for rejecting many worlds. One , scientific models only have instrumental value, one can never draw any ontological conclusion from them, or two QM as presently understood is wrong. The first assertion is just bad philosophy in my opinion and the second involves a new theory of QM for which there is currently no evidence for.
Bob Zannelli
Sean I think you should steer clear of championing the MWI. You could be left looking like a chump peddling woo. Look at work by Aephraim Steinberg et al and Jeff Lundeen et al:
http://www.physics.utoronto.ca/~aephraim/
http://www.photonicquantum.info/
Hi Sean. I’m trying to resist the urge to point out that what you say about (so-called) hidden variable theories here is somewhat misleading and unfair.
(OK, I can’t actually resist, but I’ll bracket it in parentheses… As pointed out already by EPR, QM is already nonlocal without hidden variables, so it’s misleading to suggest, as you do, that non-locality is a price one pays for introducing hidden variables. And then it’s unfair to suggest that there is no evidence for the hidden variables. As I’m sure you understand, the “hidden variables” in the dB-B pilot-wave theory are the evolving positions of the particles that compose the world we see around us. To to say that there is no evidence for the actual existence of those particles is … weird. The evidence is literally all around us all the time. Of course, other theories may attempt to explain what we see in different ways. So it’s not like just looking at a table proves conclusively that the Bohmian particles exist. But saying there is *no* evidence for them is really quite wrong. Probably it’s a result of taking the phrase “hidden variables” too literally/seriously. Bell was right when he described this terminology as “historical silliness”.)
But here’s the point I really wanted to raise. Basically the question is: what is the ontology of Everett’s theory? That is: what exactly does the theory say *exists*? To clarify what I mean, let’s start with what you say about classical mechanics: “Classical mechanics describes … a universe as a point in phase space.” That, I take it, is a kind of shorthand. What classical mechanics (of, for simplicity, let’s say, particles) describes a universe as is a collection of particles with positions and velocities. The particles live in a three-dimensional space (or a 3+1 spacetime). This is what the theory says the world is made of. The point is then that the state of this world can be *mathematically represented* as a point in phase space. But we shouldn’t confuse that abstract representation with the literal description of what, according to the theory, the world is like.
But then this focuses the question about what the world is made of according to Everettian QM. I take it that when you say things like that the state of the world is given by a wave function in Hilbert space, that is the same kind of shorthand that is involved in saying that, classically, the world is a point in phase space. So the question is: what is the literal, non-abstract way of saying what the world is like for Everettian QM, that corresponds to “particles moving around in 3D” for classical mechanics? That, to me, is the essential question that must be answered before one can make any progress on any of the other things: is what the theory says crazy or natural, should one be scandalized and refuse to accept it or instead embrace it, does Everett avoid the nonlocality that you complain afflicts hidden variable theories, etc.
If we refrain from any philosophical arguments, there is a mathematical argument why MWI is completely unnecessary. In QM we can combine two systems into a larger one by using the tensor product. In particular a quantum system can be considered with the measuring device and the environment as follows: |psi> otimes |measurement device + environment>
The tensor product is a commutative monoid, but can we upgrade it to a group? If only we would have an inverse operation… If this were possible then we can model the measurement process in a pure unitary way using the group inverse operation which will “collapse” part of the wavefunction:
|cat>\otimes |poison+environment> -> |dead cat>\otimes |poison released + new environment state>
But can we construct a group from a commutative monoid? YES WE CAN if you have an additional ingredient: an equivalence relationship. In math this is called the Grothendieck group construction. Do we have an equivalence relationship in QM? Indeed we do and it comes from a swap symmetry (http://arxiv.org/abs/1305.3594): what a quantum system can evolve unitary over here can be undone by another unitary evolution of the environment over there. Welcome to the world of envariance and quantum Darwinism. Zureks approaches the problem using physical arguments, but there is a rigorous underlying mathematical framework behind the scene.
Just like early on in special relativity people talked about “imaginary” time direction (remember ict?) because they ignore the underlying mathematical structure of the metric tensor, in QM people talk about collapse and MWI because they ignore the underlying Grothendieck group.
And by the way, in MWI there is also a pink elephant in the room: why is the split happening only after decoherence? (If not, MWI has a base ambiguity problem) Is much more natural to say that the split happens randomly regardless of decoherence. But what do you get in this case? This is no longer MWI, but GRW theory.
Bob, you’ve said that you think “non-realism” is a bad philosophy. That may be, but arguing that manipulation of mathematical symbols to match empirical results can inherently be realist is a leap I’m not willing to take. To me science is descriptive and never ontological. If you’re going to have a realist interpretation of the wavefunction, I personally would like to see some justification for doing so.
It’s funny because in normal language we would never assume our semantics are the objects, that “horse” is the same thing as a horse, we understand the word represents the concept. Yet when dealing with physics we have no problem saying that x(t) is the position of the particle or that Ψ(x, t) is the ontological state of a system. From a language point of view, assuming our symbolic representation is the ontology of the system, on the face, is absolutely absurd. Unlike formal language, the syntax of physics lines up with empirical results and even can make predictions, and perhaps this throws us off, but it’s not a reason to assume realism of our particular representation.
Sorry, the last sentence of my last comment was supposed to read, “unlike informal language.”
Dr. Carroll:
Does the MWI imply our universe is not adiabatic? If the other worlds are here now, are they still part of our universe that can come back or forbidden from interfering again (conservation of Energy)?
I am shocked to see Bohm and TIQM got 0% and Copenhagen was so high given the Afshar Experiment. Does the Afshar Experiment also falsify MWI?
I like Bohm – see title=“Photon diffraction and interference (pdf format)”. Problems with the pure Bohm are where does the pilot wave originate and how can a single photon in an experiment show interference patterns. I think TIQM holds the key to solving these issues.
Thanks Jesse Emspak.
Dustin Summy – we measure only particle interactions. Like the gravitational ether of GR. We measure only what matter does.
John Hodge
When the universe splits, how does it do this? I don’t see what law of forces allows you to simply take a bunch of stuff (ie, the universe) and clone it instantly. If the universe is billions of lightyears across and it clones instantly, doesn’t that violate the speed of light?
Am I right to assume, that humans are like Gods because, we create Universes wherever we look? Curious, how many critiques of MW go unchallenged.
Imagine that some scientists, who deny teleology or the existence of anything spiritual, believe that every human being is the Creator of trillions of independent universes!
If the vacuum could be warmed by theory as easily as the blogs tend to heat up this one, we would have multiverses everywhere. Oops! We don’t, and Schrodinger’s cat died ages ago as I confirmed on a visit to Alpbach.
Another spark to add to this MOC (figure that one out, sportsfans). Everything, reality and beyond, should behave the same whether or not “intelligent” beings are there to “observe” the outcome of events. Humans themselves do not affect the universe’s laws. An ignorant ape may observe a two-slit experiment just as well as a physicist. The outcome is the same, whether or not there is an observor. Any simple scattering experiment of particle-on-particle would generate a continuum of worlds, and this happens without an observor.
Sean, it’s hard to improve on the basic critique of the decoherence idea that Leggett made in 1987, but here are some points:
1. The basic argument from the density matrix is circular. You place observed probabilities as part of the DM as fait accompli. They are taken for granted from experimental evidence without showing how they would arise. This is not just about explaining why those particular statistics, but the whole idea of presenting “outcomes” as localized alternatives along with the wave functions that lead to them (as also criticized by Penrose.) Hence, comparing statistics under coherence and decoherence is pointless. It doesn’t show why an extended wave would remain as such under coherent conditions, but be as “classical” hits when exposed to decoherence. You have already taken the “hits” (specific exclusive interaction with yadda detector etc.) for granted, and then are just comparing different patterns of them. Indeed, we could ask, why do we observe the pattern of *hits* that display coherence in such cases at all? Instead, think that coherent waves are somehow collapsed to produce a strike-pattern corresponding to their orderly distribution, whereas incoherent waves produce a disorderly pattern of strikes, when they are “collapsed” Otherwise, it would just be orderly versus disorderly wave patterns, period.
2. Entanglement in this sense is just the consistency that if the wave ends up localized at X then it cannot be localized at Y, and so on. Well sure, but that consistency requirement is not a way to get both outcomes in any sense at all, nor explain why for example the total electric fields from alternative trajectories decided for a “Schrödinger’s Coulomb” would not both be effectively present in space, when “intereference” has nothing to do with detecting that. Indeed, the whole mistake of pretending that alternatives “wouldn’t interfere with each other” seems based on wrongly conflating the specific, optics-derived meaning of “interfere” (simply, that amplitudes add – well of course, orderly or not as may be…) with the common broad use of “interfere” as in, to affect in any way at all. And, general “interference” say of the phantom “other outcomes” would not be about being able to “undo” the disordering of the waves describing them etc, why would it – it’s a matter of actual physical interaction overall.
3. Despite the handwaving, MWI does violate conservation laws. A wave function normally describes a particle of given mass and charge etc, showing its momentum in space. For the whole of that mass etc. to be effectively found in more than one place is a completely different issue than e.g. to have a doubly-peaked WF, which still “represents” one unit of mass etc. Indeed, the process “all told” multiply instantiates a particle’s entire mass, charge, etc; each time it could be localized in alternative places. That might as well be from a mysterious “measurement” anyway. Such multiplication is not authentic continued Schrödinger evolution,
4. A certain type of MZI with three beamsplitters may be able to recombine the data of initial amplitude differences supposedly lost to decoherence. See the name link.
Well that’s all for the time being.
3. Despite the handwaving, MWI does violate conservation laws. A wave function normally describes a particle of given mass and charge etc, showing its momentum in space. For the whole of that mass etc. to be effectively found in more than one place is a completely different issue than e.g. to have a doubly-peaked WF, which still “represents” one unit of mass etc. Indeed, the process “all told” multiply instantiates a particle’s entire mass, charge, etc; each time it could be localized in alternative places. That might as well be from a mysterious “measurement” anyway. Such multiplication is not authentic continued Schrödinger evolution,
___________________
Conservation laws are about measurement. MW’s doesn’t predict energy conservation is violated during measurements, hence there is no energy conservation issue. This is a red Herring. It’s like arguing that a undetected particle can’t be multilocal because otherwise there would be a violation of conservation laws, charge, energy, momentum and angular momentum. I don’t have a clue what you’re talking about otherwise so I don’t offer any opinion.
@kashyap vasavada who said:
“Whether you bring in a conscious observer or replace him/her by a machine, the very idea of an experiment, which depends ultimately on an arbitrary human judgment (whether to do this experiment today or not) resulting in split universes, is metaphysical at best. ”
You’re assuming for no reason that Sean’s description implies that somehow *only* human-conceived experiments cause decoherence. That isn’t true, it happens constantly whenever quantum systems (which is all systems) become entangled. Conscious observers don’t need to be involved at any level. A human experiment is in this context just a way to describe a scenario that could be set up, and then describe what would happen. It’s as silly as if I were to say “If I drop this stone, it will accelerate toward the ground at 9.8m/s^2”, and you took that as me implying that humans cause gravity.
Personally most of my mental objections to EQM go away if instead of thinking of it as a universe constantly multiplying into many universes (zomg, conservation of energy!), I think of it as one universe that *divides* into subsets that can no longer interact (same amount of state as before, no CoE problem). Which, as Sean explains so well, is really the right way to think of it. Anyone comfortable with Relativity has already accepted that there are subsets of the universe whose further evolution cannot possibly affect other parts. Why is a universe divided by regions of space-time more acceptable than a universe divided by self-consistent sub-sets of states of entangled systems?
Now while on the one hand I side with everyone who says that we just don’t understand the quantum world and if we ever do, maybe then we’ll have a new picture that better explains — I will not say “makes sense” because I doubt the next theory will respect our intuition any more than QM. On the other hand I don’t think that hypothetical future possibility justifies avoiding considering the problem now. One thing I really respect about EQM is it asking “Okay, but what if QM really is a correct description, what does that imply?” Rather than sweeping the issue under the rug like Copenhagen and adding time-irreversible operations to the theory for the sole purpose of keeping QM contained inside little isolated microscopic systems and ourselves nice and classical. Even though that’s exactly what I do whenever I think about QM outside of the context of discussions like this one. 😛
Totally out of my league here, but what do you (Sean) mean that the Universe “splits”? You mean an instant copy of our Universe is created somehow, separate from our own, whenever a quantum event occurs which could have two outcomes? A copy of our entire Universe?? What mechanism has been proposed for this? Is that even science? I mean presumably these instant copies, which must spring up zillions of times per second just in my backyard, are not “knowable” to us in this timeline, right? Can a theory for how this occurs or how it could be tested, even exist? Or is this not actually science but, well, something else?
How is a new copy of our (perhaps infinite) Universe created? Where is this Universe created? At what new coordinates in the multi-verse does it spring into existence? I thought Universes were created via Big Bangs. Is this a short-cut?
You say there are fewer assumptions needed for this interpretation, that it is already part of the equations. It seems to me that this interpretation requires many, many new answers and hence new assumptions.
@Travis Norsen, who said:
“does Everett avoid the nonlocality that you complain afflicts hidden variable theories, etc.”
I do not think that is Sean’s complaint about hidden variable theories, as there are plenty non-local QM interpretations and doesn’t give that as a critique of e.g. Copenhagen. His complaint is that this unspecified variable is being added to the theory at all. The original purpose of this addition was to preserve local realism in the face of quantum entanglement, but the experimental evidence suggests that this cannot be true, and so now the “reason” to add the variable is gone.
Everett is local, though, and maybe he counts that in its favor, but it doesn’t come across that way.
@Jens who said:
“Totally out of my league here, but what do you (Sean) mean that the Universe “splits”? You mean an instant copy of our Universe is created somehow, separate from our own, whenever a quantum event occurs which could have two outcomes? ”
No, he doesn’t mean that. And realizing that’s not what is meant is I think is the biggest obstacle to accepting the theory (not accepting as undoubtedly correct, but as a valid idea that could be correct).
Think of a particle in a superposition. Do you accept that we can have an electron that is in a superposition of the states “spin-up” and “spin-down” without having two electrons, one up and one down?
Now imagine that when your apparatus interacts with the electron in a way that determines spin, the superposition does not suddenly go away, but continues with its state now entangled with the apparatus. The electron-apparatus system is now in a superposition of “spin-up measure-up” and “spin-down measure down”. Then you check the output of the apparatus, and now you are entangled with the system and it exists as a superposition of “spin-down measure-down you-read-‘down’-off-the-screen” vs the same but “up”. You don’t see a superposition of states, because each state of ‘you’ is only compatible with a subset of the states of the rest of the system. And so on with the rest of the room, the earth, etc.
In all of this nothing was “copied”, nothing was “created”. It only *didn’t* destroy half the states, they just became unavailable to you as you became entangled with the system. The universe didn’t multiply, it divided.
Bob, that is an ironically contradictory answer. If you say the conservation law doesn’t matter in this case since we can’t observe the other outcomes, then we’re not even supposed to believe in them either. It’s silly to have one standard “I can believe the other worlds ‘really exist’ because I have an ideological bent to avoid weirdness/real-randomness or whatever” but “we don’t have to worry about any absurd *consequences* of the concept since we can’t observe them”- ! And likewise to have a theoretical basis for the continuation of the states, but to ignore the theoretical basis for total mass-energy etc.
Anyway, it’s not just a legalism about being able to “get away with” the violation, where is the theoretical justification for the extra mass energy to be predicted “to exist” even in that unobservable sense? I say, if the conservation law is made irrelevant, then the concept itself is irrelevant and vacuous as well.CB
CB, that is not an answer since it just takes “entanglement” for granted without trying to figure out what makes it tick and how it fits in to observations. Nor does it give any idea whatsoever why a continuation of say, two states should effectively produce statistics of e.g. 31:69 instead of the 50:50 from having “two” streams instead of one. Entanglement is just our post-facto finding of consistency in the outcome, that if the particle is found “here” it is not also found “there.” The entanglement for two connected particles is rather different, such that if one particle is found “up” then the *other one* will also be “up” etc. But that provides no “picture” of the process or explanatory framework about what happens when the presumptive wave is localized in one spot, and how all of that mass-energy could be multiply localized in many spots, which is not at all the same issue as consistency over the whole.
Note that a superposition can indeed be found as such: superposed H and V polarization of same phase make for a diagonal polarization that is specifically passable by a filter at that orientation (that either state by itself has only a chance of passing.) Also, the simple idea of “continued states just keep going but are separate” ignores the pretense of the argument that decoherence (phase confusion) makes them non-interacting, but I explained why that argument is fallacious and there is no real reason for disorderliness of waves to constitute physical interaction as opposed to the precise definition of “interference.”
CB says:
“No, he doesn’t mean that. And realizing that’s not what is meant is I think is the biggest obstacle to accepting the theory (not accepting as undoubtedly correct, but as a valid idea that could be correct).
Think of a particle in a superposition. Do you accept that we can have an electron that is in a superposition of the states “spin-up” and “spin-down” without having two electrons, one up and one down?
Now imagine that when your apparatus interacts with the electron in a way that determines spin, the superposition does not suddenly go away, but continues with its state now entangled with the apparatus. The electron-apparatus system is now in a superposition of “spin-up measure-up” and “spin-down measure down”. Then you check the output of the apparatus, and now you are entangled with the system and it exists as a superposition of “spin-down measure-down you-read-’down’-off-the-screen” vs the same but “up”. You don’t see a superposition of states, because each state of ‘you’ is only compatible with a subset of the states of the rest of the system. And so on with the rest of the room, the earth, etc.
In all of this nothing was “copied”, nothing was “created”. It only *didn’t* destroy half the states, they just became unavailable to you as you became entangled with the system. The universe didn’t multiply, it divided.”
Thank you, CB, for your attempt at enlightening me. I’ve read your explanation 3 times now, but I must admit I still don’t understand. How could a state become “unavailable” to me unless it occurred in a separate Universe?