When physicists are asked about “parallel worlds” or ideas along those lines, they have to be careful to distinguish among different interpretations of that idea. There is the “multiverse” of inflationary cosmology, the “many worlds” or “branches of the wave function” of quantum mechanics, and “parallel branes” of string theory. Increasingly, however, people are wondering whether the first two concepts might actually represent the same underlying idea. (I think the branes are still a truly distinct notion.)
At first blush it seems crazy — or at least that was my own initial reaction. When cosmologists talk about “the multiverse,” it’s a slightly poetic term. We really just mean different regions of spacetime, far away so that we can’t observe them, but nevertheless still part of what one might reasonably want to call “the universe.” In inflationary cosmology, however, these different regions can be relatively self-contained — “pocket universes,” as Alan Guth calls them. When you combine this with string theory, the emergent local laws of physics in the different pocket universes can be very different; they can have different particles, different forces, even different numbers of dimensions. So there is a good reason to think about them as separate universes, even if they’re all part of the same underlying spacetime.
The situation in quantum mechanics is superficially entirely different. Think of Schrödinger’s Cat. Quantum mechanics describes reality in terms of wave functions, which assign numbers (amplitudes) to all the various possibilities of what we can see when we make an observation. The cat is neither alive nor dead; it is in a superposition of alive + dead. At least, until we observe it. In the simplistic Copenhagen interpretation, at the moment of observation the wave function “collapses” onto one actual possibility. We see either an alive cat or a dead cat; the other possibility has simply ceased to exist. In the Many Worlds or Everett interpretation, both possibilities continue to exist, but “we” (the macroscopic observers) are split into two, one that observes a live cat and one that observes a dead one. There are now two of us, both equally real, never to come back into contact.
These two ideas sound utterly different. In the cosmological multiverse, the other universes are simply far away; in quantum mechanics, they’re right here, but in different possibility spaces (i.e. different parts of Hilbert space, if you want to get technical). But some physicists have been musing for a while that they might actually be the same, and now there are a couple of new papers by brave thinkers from the Bay Area that make this idea explicit.
Physical Theories, Eternal Inflation, and Quantum Universe, Yasunori Nomura
The Multiverse Interpretation of Quantum Mechanics, Raphael Bousso and Leonard Susskind
Related ideas have been discussed recently under the rubric of “how to do quantum mechanics in an infinitely big universe”; see papers by Don Page and another by Anthony Aguirre, David Layzer, and Max Tegmark. But these two new ones go explicitly for the “multiverse = many-worlds” theme.
After reading these papers I’ve gone from a confused skeptic to a tentative believer. This happened for a very common reason: I realized that these ideas fit very well with other ideas I’ve been thinking about myself! So I’m going to try to explain a bit about what is going on. However, for better or for worse, my interpretation of these papers is strongly colored by my own ideas. So I’m going to explain what I think has a chance of being true; I believe it’s pretty close to what is being proposed in these papers, but don’t hold the authors responsible for anything silly that I end up saying.
There are two ideas that fit together to make this crazy-sounding proposal into something sensible. The first is quantum vacuum decay.
 When particle physicists say “vacuum,” they don’t mean “empty space,” they mean “a state of a theory that has the lowest energy of all similar-looking states.” So let’s say you have some scalar field filling the universe that can take on different values, and each different value has a different potential energy associated with it. In the course of normal evolution the field wants to settle down to a minimum of its potential energy — that’s a “vacuum.” But there can be the “true vacuum,” where the energy is really the lowest, and all sorts of “false vacua,” where you’re in a local minimum but not really a global minimum.
When particle physicists say “vacuum,” they don’t mean “empty space,” they mean “a state of a theory that has the lowest energy of all similar-looking states.” So let’s say you have some scalar field filling the universe that can take on different values, and each different value has a different potential energy associated with it. In the course of normal evolution the field wants to settle down to a minimum of its potential energy — that’s a “vacuum.” But there can be the “true vacuum,” where the energy is really the lowest, and all sorts of “false vacua,” where you’re in a local minimum but not really a global minimum.
The fate of the false vacuum was worked out in a series of famous papers by Sidney Coleman and collaborators in the 1970’s. Short version of the story: fields are subject to quantum fluctuations. So the scalar field doesn’t just sit there in its vacuum state; if you observe it, you might find it straying away a little bit. Eventually it strays so far that it climbs right over the barrier in the direction of the true vacuum. That doesn’t happen everywhere in space all at once; it just happens in one tiny region — a “bubble.” But once it happens, the field really wants to be in the true vacuum rather than the false one — it’s energetically favorable. So the bubble grows. Other bubbles form elsewhere and also grow. Eventually all the bubbles crash into each other, and you successfully complete a transition from the false vacuum to the true one. (Unless the universe expands so fast that the bubbles never reach each other.) It’s really a lot like water turning to steam through the formation of bubbles.
This is how everyone talks about the fate of the false vacuum, but it’s not what really happens. Quantum fields don’t really “fluctuate”; that’s poetic language, employed to help us connect to our classical intuition. What fluctuates are our observations — we can look at the same field multiple times and measure different values.
Likewise, when we say “a bubble forms and grows,” that’s not exactly right. What really happens is that there is a quantum amplitude for a bubble to exist, and that amplitude grows with time. When we look at the field, we see a bubble or we don’t, just like when we open Schrödinger’s box we see either a live cat or a dead cat. But really there is a quantum wave function that describes all the possibilities at once.
Keep that in mind, and now let’s introduce the second key ingredient: horizon complementarity.
The idea of horizon complementarity is a generalization of the idea of black hole complementarity, which in turn is a play on the idea of quantum complementarity. (Confused yet?) Complementarity was introduced by Niels Bohr, as a way of basically saying “you can think of an electron as a particle, or as a wave, but not as both at the same time.” That is, there are different but equally valid ways of describing something, but ways that you can’t invoke simultaneously.
For black holes, complementarity was taken to roughly mean “you can talk about what’s going on inside the black hole, or outside, but not both at the same time.” It is a way of escaping the paradox of information loss as black holes evaporate. You throw a book into a black hole, and if information is not lost you should (in principle!) be able to reconstruct what was in the book by collecting all of the Hawking radiation into which the black hole evaporates. That sounds plausible even if you don’t know exactly the mechanism by which happens. The problem is, you can draw a “slice” through spacetime that contains both the infalling book and the outgoing radiation! So where is the information really? (It’s not in both places at once — that’s forbidden by the no-cloning theorem.)
Susskind, Thorlacius, and Uglum, as well as Gerard ‘t Hooft, suggested complementarity as the solution: you can either talk about the book falling into the singularity inside the black hole, or you can talk about the Hawking radiation outside, but you can’t talk about both at once. It seems like a bit of wishful thinking to save physics from the unpalatable prospect of information being lost as black holes evaporate, but as theorists thought more and more about how black holes work, evidence accumulated that something like complementarity is really true. (See for example.)
According to black hole complementarity, someone outside the black hole shouldn’t think about what’s inside; more specifically, everything that is happening inside can be “encoded” as information on the event horizon itself. This idea works very well with holography, and the fact that the entropy of the black hole is proportional to the area of the horizon rather than the volume of what’s inside. Basically you are replacing “inside the black hole” with “information living on the horizon.” (Or really the “stretched horizon,” just outside the real horizon. This connects with the membrane paradigm for black hole physics, but this blog post is already way too long as it is.)
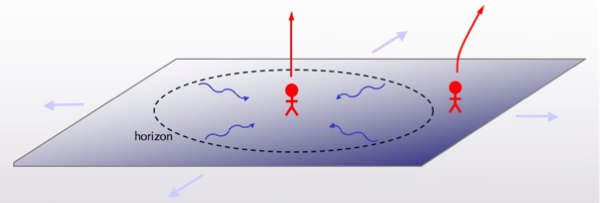
Event horizons aren’t the only kind of horizons in general relativity; there are also horizons in cosmology. The difference is that we can stand outside the black hole, while we are inside the universe. So the cosmological horizon is a sphere that surrounds us; it’s the point past which things are so far away that light signals from them don’t have time to reach us.
So then we have horizon complementarity: you can talk about what’s inside your cosmological horizon, but not what’s outside. Rather, everything that you think might be going on outside can be encoded in the form of information on the horizon itself, just like for black holes! This becomes a fairly sharp and believable statement in empty space with a cosmological constant (de Sitter space), where there is even an exact analogue of Hawking radiation. But horizon complementarity says that it’s true more generally.
So, all those pocket universes that cosmologists talk about? Nonsense, say the complementarians. Or at least, you shouldn’t take them literally; all you should ever talk about at once is what happens inside (and on) your own horizon. That’s a finite amount of stuff, not an infinitely big multiverse. As you might imagine, this perspective has very deep consequences for cosmological predictions, and the debate about how to make it all fit together is raging within the community. (I’m helping to organize a big meeting about it this summer at Perimeter.)
Okay, now let’s put the two ideas together: horizon complementarity (“only think about what’s inside your observable universe”) and quantum vacuum decay (“at any point in space you are in a quantum superposition of different vacuum states”).
The result is: multiverse-in-a-box. Or at least, multiverse-in-an-horizon. On the one hand, complementarity says that we shouldn’t think about what’s outside our observable universe; every question that it is sensible to ask can be answered in terms of what’s happening inside a single horizon. On the other, quantum mechanics says that a complete description of what’s actually inside our observable universe includes an amplitude for being in various possible states. So we’ve replaced the cosmological multiverse, where different states are located in widely separated regions of spacetime, with a localized multiverse, where the different states are all right here, just in different branches of the wave function.
That’s a lot to swallow, but hopefully the basics are clear. So: is it true? And if so, what can we do with it?
Obviously we don’t yet know the answer to either question, but it’s exciting to think about. I’m kind of inclined to think that it has a good chance of actually being true. And if so, of course what I’d like to do is to ask what the consequences are for cosmological initial conditions and the arrow of time. I certainly don’t think this perspective provides an easy answer to those questions, but it might offer a relatively stable platform from which definite answers could be developed. It’s a very big universe, we should expect that understanding it will be a grand challenge.
#50 — Why should anyone accept that an electron goes through two slits at once, when there is a reasonable explanation for the electron to go through only one slit at a time while building up an interference pattern? It sounds like giving an unrealistic explanation the same status as a realistic explanation. There is no reason in nature why an electron, a material body, should be in two different places at the same time. The wave function is a statistical ensemble. I don’t think what I am describing is the “pilot wave” but I’m not sure how you would equate a collective motion with the statistical ensemble that gives the probability for each location that the electron is there.
Ok, so Just to clarify things as I see them. These questions are still being explored today by Extra solar and interdimensional travelers who are expanding the research of the theories we are postulating today. But i guess it’s good we think about things and questions now so they can be answered in the future. Which technically is now and “they” are from what you might call the future. “They” go back and forth conducting timeline specific research on these and more advanced theories. It’s a never ending process of discovery, and it drives all societies to step forward and look for answers to their questions; what ever they may be.
#51,
Because trying to maintain only a single electron results in a mathematically more complicated theory, and requires disturbances in it to propagate faster than light (and hence backwards in time). It was while arguing about the pilot wave theory that Bell came up with his inequalities, to show that any interpretation that claimed a single definite classical state throughout had to be non-local (i.e. involve faster-than-light). You also have to introduce a new field, and an interaction law between the electron and this field, and new physics to describe what happens to the field when you observe the electron.
Or to put it another way, the pilot wave theory is also an unrealistic explanation – it’s just unrealistic in a different way.
The basic problem is that our intuitions about the way the world is have been built up around classical physics, so anything else looks weird and unrealistic. Even the physicists developing it struggled with the idea that the world we lived in wasn’t really classical. Bohr’s Copenhagen interpretation tried to fudge it by saying it was quantum up to the moment of observation when it “collapsed” into a classical outcome. DeBroglie and Bohm tried to say it was a classical world that got pushed around by this quantum thing when nobody was looking. Einstein tried to say that it was classical throughout, it was just there were interactions and hidden states we hadn’t yet discovered that gave the illusion that it was quantum. Others said the question was meaningless, QM didn’t tell you how the world really works, it only told you the outcome of experiments, so you should “just shut up and calculate”.
Everett simply pointed out that if the world was quantum at every level, that what we would see as quantum objects ourselves would be precisely a classical looking world. From the outside, it looks like a wave, but from the inside it should look like billiard ball particles bouncing off one another.
So he said, why invent two entirely separate physics, a quantum world and a classical world, and then tie yourself in non-local knots trying to glue the two together, when a purely quantum world already does the job?
The quantum picture predicts everything we observe, except that it also predicts a bunch of other stuff should be going on where we can’t ever see it. Since we can’t see it, we’re inclined not to believe it exists. Trying to come up with a mechanism by which it can disappear as required has led to a huge amount of debate. But we’ll never know, because we can’t see it to check.
If you’re saying that your theory is your own idea, rather than the DeBroglie-Bohm theory, then you’ll have to develop the details some more for anyone to offer an opinion. You’ll need to come up with an equation for the electron-field interaction, another for the propagation of the field, say how it electrons/fields interact with other charged particles, and show how it explains experiments like EPR, Bell’s inequalities, the delayed choice quantum eraser, and so on. But at the end of the day, it won’t prove that MWI is wrong, only that it’s not the only option. You have a choice.
#53 — I may not understand what you mean by “trying to maintain only a single electron” (or the sentence), but I take it you mean that the real path of an electron is not calculable without taking into account its other possible paths which are not real. I would describe that as a kind of half-entanglement. It implies acknowledgement of a previous history which cannot be traced (even under the most controlled experiment), but whose influence does not diminish: if the unlikely previous history did happen, then the electron will take that unlikely path. So the probability is really accounting for unknown previous history. When electrons can be tracked, they can be seen to become “fully” entangled (spin up-down) so that neither electron can be considered without the other. But I don’t think the MWI-multiverse idea is anything to do with that.
However a wave is a wide area “disturbance in the field” (of whatever kind). It may be treatable as following a path but its effect is over a large area. An electron is a spatially extended but tiny and local material entity, and it doesn’t make sense to demand, because of the math, that an electron in physical reality can be in two places at once (or act like a wave). One of the places is not a real place (it is an unreal path). The interference pattern, which is a statistical pattern independent of time and space (provided the equipment is identical), is not due to the electron being in two places at once when no one is looking. I do not believe anyone who thinks this over for even a short time will say it is reasonable for an electron to be in two places at once. The fact that this is demanded by the math does not mean it is demanded by physical reality. It is an indication that the physical reality is not understood. The task then is to find out a physical mechanism that can reproduce the q.m. results. But this has not been done for 100 years – entanglement seems to have mesmerized people, they forget there is no realistic spatially extended electron model. Such a model still must recognize entanglement, but gives the possibility of ending the fruitless debate over different interpretations, because it will give the local realistic hidden variable machinery by which the q.m. results are found. I have indeed worked on this model and am convinced by it. However, there should be “real scientists” looking down this path. A realistic electron model will undoubtedly clear up the problem of interpretation. I am not saying the world can go back to being “classical”, but it can come to an understanding of q.m. results that doesn’t involve electrons being in two places at once, many worlds, multiverses, etc. Describing a triaxially rotating sphere (of rotating space) rotating at c at a radius from the proton (also a sphere) between the classical and Bohr radii (separated from each by inverse alpha), and showing how that sphere of rotating space will move when there is a disturbance in the field its vicinity, will lead to a new and more explanatory model for atomic and nuclear systems than the SM. And eventually the interpretational problems of quantum mechanics will disappear.
Is physics turning into some kind of a joke? I find it comical how physicists follow intellectual fashions and subscribe to occult belief systems like multiverse and string theory, while claiming to hold the intellectual high ground as the arbiters of ultimate truth. Please be aware of your absurd hubris! To the unindoctrinated, multiversal quantum theory sounds no more credible than ancient mystical ideas about astral planes and reality-as-illusion.
More than a century ago, the great philosopher Nietzsche predicted that science would eventually turn its lens upon itself and self-destruct, and it seems that yet again he was prophetic!
I published a paper some years ago (arXiv:gr-qc/0611098 and references therein)
proposing another kind of parallel (or antiparallel, with reversed
time evolution) worlds. The main idea was to use the
direct sum of weakly interacting quantum fields to represent
parallel worlds, as distinguished from the usual procedure
of taking direct products of fields for the various particle
families. One observable correlate that I proposed
was that a huge cancellation of vacuum energies occurs
between our world and a hypothetical weakly connected one with a reverse
evolution in time. Another was that of the existence of gravitational waves converging
on future events in space-time.
@55: No, it’s no kind of joke, and it’s not mysticism. And Nietzsche didn’t really understand science, certainly not 21st-century science.
These “intellectual fashions” of physicists are basically proposals for possible ways of understanding the theories which developed to solve real problems with real empirical observations. If you go through a text like Roger Penrose’s The Road to Reality and manage to stomach all of the mathematics (good luck with that), and brush up on the history behind the development of quantum theory, relativity theory, and quantum theory, you will see that it all rests on real observations and real scientific problems which those observations threw up. It’s just that trying to talk about these subjects without using the math you will find in Penrose’s book, as Prof. Carroll is very courageously trying to do, makes it sound rather weird, perhaps.
These remark also apply to “Liberalism”‘s attempt to equate physics and religion.
No predictions, no science.
OK JonJ, but what empirical observations is multiverse/string theory based on, and what testable predictions can it make? And how is your advice distinguishable from an occultist telling me that if I study various magical texts and follow certain esoteric practices for several years, I will be able to understand his mystical teachings? I think the physics priesthood is facing a huge crisis if the best they can come up with going forward is untestable, mystical-theological string/multiverse theories, and by extension the entire scientific paradigm may be in deep trouble!
#59,
For the empirical observations, you’ll need to follow JonJ’s advice and get some good books on the experimental history of quantum mechanics. Or better, do a course where you get to try it out for yourself in the lab.
But to answer your question about how they are distinguishable to a layman who doesn’t want to learn the maths – the answer is that physics works, and occultism doesn’t. You can play Sonic the Hedgehog on the products of quantum physics, which you can go out and buy in the shops. If it didn’t work, it would soon be noticed.
Other than that, your question makes as much sense as asking how you can tell if Japanese Haiku make sense when you don’t speak Japanese. I have met people who pretended to be speaking Japanese when they were actually making up nonsense syllables, but the basic test is to drop them in the middle of Japan and see if they can make their way. Even if you don’t know what they’re saying, you can see whether or not it works. Complaining that they all sound the same to you is not an effective test.
OK please demonstrate to me the multiverse in action, or cosmology, or string theory. I’m not questioning whether quantum effects are real, I’m questioning whether these multiverse and cosmological ideas are even science, as opposed to some kind of non-empirical mathematical theology.
The physical world is far too messy and uncooperative for our celebrity Platonists.
They like to wax eloquent in the purely abstract.
The better to avoid that crackpot naysayer: nature.
Sean,
Curious to know what you think about ‘T Hooft’s latest paper
http://arxiv.org/abs/1104.4543
“I’m not questioning whether quantum effects are real”
Jolly good. The MWI is simply the assertion that quantum effects are really real, and apply to the entire universe, us included. So if you’re not questioning it, we’re done, right?
(Oh, and string theory is an entirely separate matter, for which the fundamental basis is that point particles don’t work, because an inverse square law gives severe mathematical difficulties at zero radius. If points don’t work, there has to be an extended structure, and the simplest alternative to a point is a string. If you want to disprove it, all you have to do is explain how the inverse square law operates at the electron’s actual location.)
#64 Nullius in Verba (at the risk of disrupting the current conversation), if you decide that spatial extension is necessary, a string does not seem such a likely candidate since it has only two dimensions, is that not right? Is this due to the demands of the math? A more likely spatially extended particle would be a sphere, and it has recently been discovered that an electron is indeed an almost perfect sphere. In the model I follow, the sphere besides rotating also oscillates — breathes in and out. If your requirement of an explanation of the inverse square law is a generally recognized requirement, the charge would be spread out over the entire sphere, and then you would have a charge per square meter. As the sphere oscillates (its frequency change is equal to the frequency of 13.6 eV), I do not know how you would handle that mathematically. In this model the electron sphere surrounds the proton sphere, and when they are in that system there is no force between them. When they are separated (the electron is ejected from its orbital space) the electromagnetic force emerges. (And an atom is rotating spheres, all the way in!)
#65,
Disrupt away! The other conversation wasn’t going anywhere.
While string theory did start with string, it has extended to include higher-dimensional entities – membranes and n-branes and so on, in what is now known as M-theory. So yes, they’ve considered more than just strings.
The articles about the electron being a sphere are a bit of a simplification – what they’re actually saying is that the charge distribution so far as we can measure it is spherical (the electric dipole moment is close to zero – the extent to which the charge is spread out along an axis), although it is not expected to be exactly so, as there are influences of asymmetric physics that are expected to influence it. However, this is measured at a scale far, far larger than strings are thought to be, so it doesn’t really say much about string theory, at least, not directly.
Supposing the electron to be a uniformly charged sphere, you might like to think about what force holds it together against the self-repulsion of the charge. Is the charge uniform throughout the volume, or concentrated on the surface? Is it flexible or rigid? Is the charge fixed in the solid body, or can it flow? What effect does the spin of the electron have – is it like a metal sphere spinning, or something else? When an electron and positron collide, what happens to the charge? And how can you reliably distinguish between all these options?
The size of the electron is usually thought of as being much smaller than a proton, although in atoms its wavefunction is spread out over a larger space. Experiments in colliders find the inverse square relationship for the nucleus breaks down at a certain point – when the particles come into physical contact – but electrons have been pushed together much closer with no breakdown of the inverse square to suggest such a limit has been reached. You would need to think about why that would be.
Try reading Penrose’s book, as JonJ recommended. It would give you the background in what has gone before, so you will know what sort of things to try, and all the many features your theory will need to explain.
“The MWI is simply the assertion that quantum effects are really real, and apply to the entire universe, us included.”
To which I say: who cares? If there are no testable consequences of this model, what possible difference does it make? I find it amusing that people are actually willing to pay the salaries of these modern day theologians so they can travel around the world to conferences discussing the scientific equivalent of how many angels can fit on the head of a pin. Enjoy it while it lasts, because I suspect this secular priesthood’s days are numbered…
#66, I have read “The Road to Reality”, as thoroughly as possible given no physics and little math training. It was very useful but, naturally with quantum mechanics, unphysical when it came to the processes. But it explained a lot, more than any other book I have read (I have also read “Six Easy Pieces” and “QED” and they are also first rate).
You have given some meaty suggestions and questions, which I appreciate very much.
“Supposing the electron to be a uniformly charged sphere . . . what force holds it together against the self-repulsion of the charge.”
I think the first thing to address is “charge”. I believe the actions and reactions due to what is called charge are a spatial phenomenon. The proton and electron when separated are connected by some motion of space, some rotational alignment (wide area in effect). That is the electromagnetic effect. It does not exist within an electron-proton system. However, the “charge” can be deconstructed into its physical units, and there is then a current totalling the elementary charge. The current is timed at one second. So one second of current totalling the elementary charge is part of the structure of the system. The “energy” gives a frequency in the Planck equation, etc. So the electron is a massless current — and for experiment it can be in amperes, etc. The current in this model is simply rotating space. In other words, in this model there is no hyphenated spacetime-energy complex. Spacetime is energetic; energy is spacetime, which in turn is motion. Without the energy then the field disappears just as in general relativity. So “rotating space” is spatially oriented motion, or spatially oriented energy.
So there is no force holding a rotating charge against its self-repulsion. What there is, in this model, is a force creating and maintaining the rotating sphere. This force takes the form of a (constant) acceleration due to change of direction. It dissipates the energy of a (the) really fundamental universal physical field. This energy takes the form of pressure against both the interior and exterior surfaces of the sphere; the pressure is relieved by rotation, but the rotation must be on the equivalent of three axes in order to absorb pressure from every direction.
This means then that there is a “vacuum pressure” which in this case creates (in a creation scenario) the massive bodies of the universe. If there is no e.m. force between the system electron and proton (i.e. when they are together as a hydrogen atom), similarly there is no force between the multiple spheres that exist in heavy atoms. But there is a constant and complex action-reaction sequence among the relative motions of each sphere that strikes me at the moment as hopelessly complex (even uncalculable). Anyway the idea is that nuclear radiation is a net effect of the juggling of the ever less stable assemblies of rotating and oscillating spheres (as the atoms get bigger). An “electron” emerges from the nucleus because that is the form that emerges after all the juggling is done. (Maybe the juggling would be transfer of momentum.) And the bottom line is that the really fundamental universal physical field has a limit of available energy, and it is possible to create systems that exceed this limit for short periods of time (like a neutron) by juggling the motions of the intermediate fields (between spheres). So there is a kind of “vacuum expectation value” here but I’m not sure it is a q.m. kind.
This scenario is then for a constantly “powered” universe.
“What effect does the spin of the electron have – is it like a metal sphere spinning, or something else? When an electron and positron collide, what happens to the charge? And how can you reliably distinguish between all these options?”
In this model the spin determines the path of the electron through a magnetic field, just as “normal”. The main feature of this model is that the electron rotates on two of three possible axes. One of the axes is the “electric” axis; the other is one of two possible “magnetic” axes. Which is electric and which is magnetic, no one can ever know, because it is a spatial orientation process — there is a spatial motion “key” which is equivalent of the recognition of “charge”. But note that the electron can take one of two possible magnetic axes — there are three possible axial relations. I believe this will give the superposition style q.m. statistical result. The spin here is real, actual, physical rotation. The electron is not a tiny dot but a rotating sphere with a radius between the classical and Bohr radius. So it is a large region of space. The proton is eighteen hundred times smaller. The inertial mass of the bodies is due to the spatial origin of their rotation. Space is moving toward the body from every direction (the external field is infinite in space but the internal field is finite). That net motion of space toward it holds the body in place.
When an electron and positron collide, their “charge” (which is really a temporary phenomenon) is extinguished. I do not know what form the resulting energy will take. And in general I don’t know whether this model can be tested, but it does give the electron magnetic moment to seven calculator decimal places, with no spin-g factor. That to me is almost like “proof”, but other people may not agree.
How the inverse square law would apply to a sphere like the one here, I don’t know. But the current is simply a spatial current – a motion of space – and as well as forming a rotating spherical structure it also oscillates in and out, and this is due to the temporary depletion and renewal of the field in the vicinity. It seems to me this means it cannot be a perfect sphere. Now try to imagine this rotating and oscillating sphere responding to pressure variations arriving in waves at its surface. This would be “electromagnetic radiation” and would have two pressure components or vectors, at 90 degrees. The response of the electron to the “applied energy” depends on the direction and internal structure of the wave, and also on the specific rotational pattern of the electron. So it is pretty well uncalculable. But somehow some specific form of spatial disturbance will be retransmitted by the electron, and it will bear the mark both of the electron’s rotation and of the previous wave’s “polarity”. Can this be visualized or animated? I don’t know, but it seems to me somebody should take it on. (It won’t be me, I tried it and went mad.)
Instinctively I like it Saun.
I too am troubled by the increasingly “metaphysical”, almost theological trends in Mathematical Physics.
Anything that grounds physics more-firmly in observable reality gets my interest at least, if not my support.
Empiricists need to take back physics from the Platonists.
The mystics need to driven back to the Math Dept where they belong.
Physics belongs among the empirical sciences.
The deeper question is: whether there are actual laws of Nature that impose this rigorous empiricism on us? Actual discoverable laws? One feels there could well be.
How could you have an philosophically-complete physics that didn’t have its rigorous empiricism built in to its axioms?
A nature that isn’t profoundly closed is a nest of spooks.
What is known must be a subset of what is knowable. Must be. Couldn’t be anything else.
MWI is not at heart consistent with the Born probability rule. Simply, MWI has as many “branches” as choices, but the BR requires squared amplitudes for e.g. superpositions with unequal amplitudes. After say 100 trials, counting up branches gives 50/50 type frequentist statistics, which is wrong if you need other ratios.
Pingback: When the multiverse and many-worlds collide « physics4me
Joel Goldsmith: “God is the substance of all being.”
Individual being-awareness is God-Being-Awareness.
Each of us already being all of single entire unified creative fractal
hyperinfinity, always shimmering along all possible corridors of
meaning, the putative present, the constantly evolving pasts, the
entire manifold of ever increasingly fabulous futures, with “sideways”
causality among all streams of probable history world lines,
“vertical” causality among an ascending (Georg Cantor) infinite
hierarchy of levels of infinity realms — every most infinitesimal
point in intimate rapport with every other — jus’ ussens, folks…..
prodigious smidgons of nonduality…..
CSICON — Murray’s Law — Eternal Exponential Expansion of Science:
Rich Murray 1997.04.05, 2001.06.22, 2011.01.03
http://rmforall.blogspot.com/2011_01_01_archive.htm
Tuesday, January 3, 2011
[ at the end of each long post, click on Older Posts ]
http://groups.yahoo.com/group/rmforall/message/102
[ you may have to Copy and Paste URLs into your browser ]
Rich Murray CSICON April 5 1997
Communion for the Subjective Investigation of Claims of the Normal
@72,
I’d rather assume the Born Rule than assume a Collapse of the Wave Function. At least there are some decent arguments that the MWI is no worse off than classical mechanics when it comes to probability. There are no decent arguments that the Wave Function actually collapses — and as a result it is an assumption too far in my view.
Pingback: Decoherence « Eikonal Blog
Very nice article . It leaves some loose ends but on the other hand both physics itself and our perception of the universe will always leave some holes to fill considering that:
a) the universe is not intrinsically finite, it is only observed empirically to be finite – the idea of finite is first of all a man made concept, therefore the universe cannot ever be said to have an intrinsic ‘boundary’ other than the obvious observation-based one;
b) we constantly, continuously and collectively modify the universe by our actions and our words (both of which are based and dependent on perception and imagination). However, any action can be infinitesimally divisible on the axis of (time-dependent and time-independent) perception therefore the entirety of the universe will be unknown by at least a finite and infinitesimally small amount;
c) our perception, reasoning, feeling and acknowledgment of the universe and ourselves is constantly expanding both in x,y,z,t coordinates, in microscopic and macroscopic depth and in all the rest of inner dimensions with each successive generation as is our extended perception (technologically aided one). However, even if we could in a very very distant future let’s say, empirically observe infinity, we cannot empirically observed the middle of a star or even approach it past a certain distance, unless we’re either in a ‘moving black hole’, in an impermeable protective bubble or the observation itself is purely imaginary (or unconscious);
d) our ability to measure is intrinsically linked to our ability to observe (which is itself exponentially related to our ability to observe with an aid). The exponential curve of perception would be even steeper if our technological aids could build their own aids, but we still have to wait a little before that happens – although programming software programmers (meta-programming) or programming genes that program genes (meta-genetics) are a couple of inches away. however, because of this intrinsic link and because of point a), the possibility of a microscopic bottom or a macroscopic upper boundary of the universe is null, a boundary of this sort can only exist conceptually.
This being said, it seems almost obvious that the universe itself is not finite and that our perception of it fades to black (or to nothing, to be more precise). Now what exactly is this nothing? Let me ask you one thing. Imagine the Big Bang. If it ever happened, then that means that all the astral bodies in the universe should be radially diverging from a point of origin. Any physical emergence has a point of ignition, a center, a source if you will, even in swarm behavior based emergence (consider how a predator spreads a bank of fish, the fish radially diverge from the predator). Considering that any measurement that is not continuous, real-time and constant (a flux) is an incomplete measurement, and considering that everything is constantly moving, has anybody tried to measure if the astral bodies in the universe that we have managed to observe so far are indeed radially divergent from this hypothetical point Origin (i.e. the source of the Big Bang)? To make such a measurement, one would need to continuously measure the motion of the centers of gravity of 3 (any 3) galaxies over a certain period of time. Let’s say that we measure Galaxies A, B and C.
Consider the 3 measured distances A to B, B to C and A to C. They form a triangle, obviously. By observing how A to B varies in relationship to B to C, how B to C varies in relationship to A to C and how A to C varies in relationship to A to B while keeping in mind that they are points on the same expanding three-dimensional wave of force radiating from the hypothetical Big Bang, one can find out if a hypothetical CENTER of the universe (i.e. the source of the Big Bang) exists. In simpler words, 3 Galaxies, when measured and observed from their centers of mass, form a triangle. Any triangle, in a three-dimensional wave of force expanding from the Big Bang (an expanding sphere) scales over time, except if all three points are in the center of the radiating wave of force in which case the triangle itself doesn’t exist. Any scaled triangle, in three-dimensional space, maintains its angles at the same values. Therefore if the Big Bang happened, any 3 Galaxies that we continuously, coherently and constantly (over time) measure according to the rules above, will maintain the angles of their composite triangle. If they don’t, the Big Bang never happened. If the Big Bang did not happen, then Universal entropy does not exist and the Universe can be thought of as an application of Bernoulli’s rule of thermal agitation on a homogeneously stochastic space filled with hydrogen atoms and that presents local emergence (i.e. other chemical elements). If this measurement yields non-consistent results (i.e. the angles do not remain constant) one could either hypothesize dark matter or, better yet, ask how did the hydrogen atom appeared and what exactly it is to begin with. Furthermore, consider that we can simulate our own galaxy (even the past position of our planet in our galaxy, relative to its center of mass), and that we can calculate the distances between the 3 galaxies to be measured and make the necessary calculations and corrections so that the measurements are time consistent for all of the galaxies involved in relationship to one another (i.e. they are at the same position in objective time, for all 3 measured galaxies).
Now please consider everything written so far. It is safe to assume that hydrogen is the oldest element in the universe other than nothing, due to its huge, observed proportions in physical reality. Consider that carbon dating cannot measure the age of hydrogen, consider that the big bang can be epistemologically and ontologically questioned and verified through the measurement described above, consider the supposed existence of dark matter.and consider the following: whatever “oldest astral body” we will find, there will always be the possibility of an even older astral body outside of our current area of perception, because of the fact that the origin point of the big bang cannot be defined (due to the posited existence of dark matter). Therefore even if we could measure the age of hydrogen in the observable universe, the constant “even older” astral body would have at least one even older hydrogen atom in its vicinity. Does the age of the universe depend on human perception and human measurement as and extension of human observation and perception? Is what is believed to be the Big Bang the birth of our empirical perception of the universe?
Moreover, all current ways to measure the age of hydrogen are flawed because they are based on the supposition of the Big Bang: http://adsabs.harvard.edu/full/1969ARA&A…7…39K. They are all distribution based, and take into account the Big Bang as a given when the Big Bang itself was never proven empirically through the measurement described above.
Considering everything written so far, and considering that everything can be described geometrically, including the wave function, including physical reality, including every possible force, is it possible that reality is the “virtual” emergence of a hydrogen based, self sustainable, autopoietic universal processor?