When physicists are asked about “parallel worlds” or ideas along those lines, they have to be careful to distinguish among different interpretations of that idea. There is the “multiverse” of inflationary cosmology, the “many worlds” or “branches of the wave function” of quantum mechanics, and “parallel branes” of string theory. Increasingly, however, people are wondering whether the first two concepts might actually represent the same underlying idea. (I think the branes are still a truly distinct notion.)
At first blush it seems crazy — or at least that was my own initial reaction. When cosmologists talk about “the multiverse,” it’s a slightly poetic term. We really just mean different regions of spacetime, far away so that we can’t observe them, but nevertheless still part of what one might reasonably want to call “the universe.” In inflationary cosmology, however, these different regions can be relatively self-contained — “pocket universes,” as Alan Guth calls them. When you combine this with string theory, the emergent local laws of physics in the different pocket universes can be very different; they can have different particles, different forces, even different numbers of dimensions. So there is a good reason to think about them as separate universes, even if they’re all part of the same underlying spacetime.
The situation in quantum mechanics is superficially entirely different. Think of Schrödinger’s Cat. Quantum mechanics describes reality in terms of wave functions, which assign numbers (amplitudes) to all the various possibilities of what we can see when we make an observation. The cat is neither alive nor dead; it is in a superposition of alive + dead. At least, until we observe it. In the simplistic Copenhagen interpretation, at the moment of observation the wave function “collapses” onto one actual possibility. We see either an alive cat or a dead cat; the other possibility has simply ceased to exist. In the Many Worlds or Everett interpretation, both possibilities continue to exist, but “we” (the macroscopic observers) are split into two, one that observes a live cat and one that observes a dead one. There are now two of us, both equally real, never to come back into contact.
These two ideas sound utterly different. In the cosmological multiverse, the other universes are simply far away; in quantum mechanics, they’re right here, but in different possibility spaces (i.e. different parts of Hilbert space, if you want to get technical). But some physicists have been musing for a while that they might actually be the same, and now there are a couple of new papers by brave thinkers from the Bay Area that make this idea explicit.
Physical Theories, Eternal Inflation, and Quantum Universe, Yasunori Nomura
The Multiverse Interpretation of Quantum Mechanics, Raphael Bousso and Leonard Susskind
Related ideas have been discussed recently under the rubric of “how to do quantum mechanics in an infinitely big universe”; see papers by Don Page and another by Anthony Aguirre, David Layzer, and Max Tegmark. But these two new ones go explicitly for the “multiverse = many-worlds” theme.
After reading these papers I’ve gone from a confused skeptic to a tentative believer. This happened for a very common reason: I realized that these ideas fit very well with other ideas I’ve been thinking about myself! So I’m going to try to explain a bit about what is going on. However, for better or for worse, my interpretation of these papers is strongly colored by my own ideas. So I’m going to explain what I think has a chance of being true; I believe it’s pretty close to what is being proposed in these papers, but don’t hold the authors responsible for anything silly that I end up saying.
There are two ideas that fit together to make this crazy-sounding proposal into something sensible. The first is quantum vacuum decay.
When particle physicists say “vacuum,” they don’t mean “empty space,” they mean “a state of a theory that has the lowest energy of all similar-looking states.” So let’s say you have some scalar field filling the universe that can take on different values, and each different value has a different potential energy associated with it. In the course of normal evolution the field wants to settle down to a minimum of its potential energy — that’s a “vacuum.” But there can be the “true vacuum,” where the energy is really the lowest, and all sorts of “false vacua,” where you’re in a local minimum but not really a global minimum.
The fate of the false vacuum was worked out in a series of famous papers by Sidney Coleman and collaborators in the 1970’s. Short version of the story: fields are subject to quantum fluctuations. So the scalar field doesn’t just sit there in its vacuum state; if you observe it, you might find it straying away a little bit. Eventually it strays so far that it climbs right over the barrier in the direction of the true vacuum. That doesn’t happen everywhere in space all at once; it just happens in one tiny region — a “bubble.” But once it happens, the field really wants to be in the true vacuum rather than the false one — it’s energetically favorable. So the bubble grows. Other bubbles form elsewhere and also grow. Eventually all the bubbles crash into each other, and you successfully complete a transition from the false vacuum to the true one. (Unless the universe expands so fast that the bubbles never reach each other.) It’s really a lot like water turning to steam through the formation of bubbles.
This is how everyone talks about the fate of the false vacuum, but it’s not what really happens. Quantum fields don’t really “fluctuate”; that’s poetic language, employed to help us connect to our classical intuition. What fluctuates are our observations — we can look at the same field multiple times and measure different values.
Likewise, when we say “a bubble forms and grows,” that’s not exactly right. What really happens is that there is a quantum amplitude for a bubble to exist, and that amplitude grows with time. When we look at the field, we see a bubble or we don’t, just like when we open Schrödinger’s box we see either a live cat or a dead cat. But really there is a quantum wave function that describes all the possibilities at once.
Keep that in mind, and now let’s introduce the second key ingredient: horizon complementarity.
The idea of horizon complementarity is a generalization of the idea of black hole complementarity, which in turn is a play on the idea of quantum complementarity. (Confused yet?) Complementarity was introduced by Niels Bohr, as a way of basically saying “you can think of an electron as a particle, or as a wave, but not as both at the same time.” That is, there are different but equally valid ways of describing something, but ways that you can’t invoke simultaneously.
For black holes, complementarity was taken to roughly mean “you can talk about what’s going on inside the black hole, or outside, but not both at the same time.” It is a way of escaping the paradox of information loss as black holes evaporate. You throw a book into a black hole, and if information is not lost you should (in principle!) be able to reconstruct what was in the book by collecting all of the Hawking radiation into which the black hole evaporates. That sounds plausible even if you don’t know exactly the mechanism by which happens. The problem is, you can draw a “slice” through spacetime that contains both the infalling book and the outgoing radiation! So where is the information really? (It’s not in both places at once — that’s forbidden by the no-cloning theorem.)
Susskind, Thorlacius, and Uglum, as well as Gerard ‘t Hooft, suggested complementarity as the solution: you can either talk about the book falling into the singularity inside the black hole, or you can talk about the Hawking radiation outside, but you can’t talk about both at once. It seems like a bit of wishful thinking to save physics from the unpalatable prospect of information being lost as black holes evaporate, but as theorists thought more and more about how black holes work, evidence accumulated that something like complementarity is really true. (See for example.)
According to black hole complementarity, someone outside the black hole shouldn’t think about what’s inside; more specifically, everything that is happening inside can be “encoded” as information on the event horizon itself. This idea works very well with holography, and the fact that the entropy of the black hole is proportional to the area of the horizon rather than the volume of what’s inside. Basically you are replacing “inside the black hole” with “information living on the horizon.” (Or really the “stretched horizon,” just outside the real horizon. This connects with the membrane paradigm for black hole physics, but this blog post is already way too long as it is.)
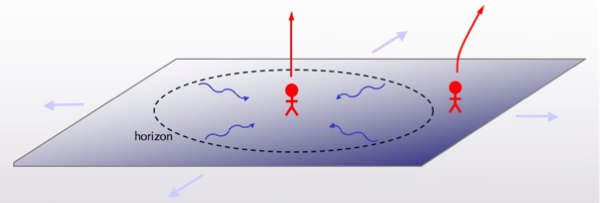
Event horizons aren’t the only kind of horizons in general relativity; there are also horizons in cosmology. The difference is that we can stand outside the black hole, while we are inside the universe. So the cosmological horizon is a sphere that surrounds us; it’s the point past which things are so far away that light signals from them don’t have time to reach us.
So then we have horizon complementarity: you can talk about what’s inside your cosmological horizon, but not what’s outside. Rather, everything that you think might be going on outside can be encoded in the form of information on the horizon itself, just like for black holes! This becomes a fairly sharp and believable statement in empty space with a cosmological constant (de Sitter space), where there is even an exact analogue of Hawking radiation. But horizon complementarity says that it’s true more generally.
So, all those pocket universes that cosmologists talk about? Nonsense, say the complementarians. Or at least, you shouldn’t take them literally; all you should ever talk about at once is what happens inside (and on) your own horizon. That’s a finite amount of stuff, not an infinitely big multiverse. As you might imagine, this perspective has very deep consequences for cosmological predictions, and the debate about how to make it all fit together is raging within the community. (I’m helping to organize a big meeting about it this summer at Perimeter.)
Okay, now let’s put the two ideas together: horizon complementarity (“only think about what’s inside your observable universe”) and quantum vacuum decay (“at any point in space you are in a quantum superposition of different vacuum states”).
The result is: multiverse-in-a-box. Or at least, multiverse-in-an-horizon. On the one hand, complementarity says that we shouldn’t think about what’s outside our observable universe; every question that it is sensible to ask can be answered in terms of what’s happening inside a single horizon. On the other, quantum mechanics says that a complete description of what’s actually inside our observable universe includes an amplitude for being in various possible states. So we’ve replaced the cosmological multiverse, where different states are located in widely separated regions of spacetime, with a localized multiverse, where the different states are all right here, just in different branches of the wave function.
That’s a lot to swallow, but hopefully the basics are clear. So: is it true? And if so, what can we do with it?
Obviously we don’t yet know the answer to either question, but it’s exciting to think about. I’m kind of inclined to think that it has a good chance of actually being true. And if so, of course what I’d like to do is to ask what the consequences are for cosmological initial conditions and the arrow of time. I certainly don’t think this perspective provides an easy answer to those questions, but it might offer a relatively stable platform from which definite answers could be developed. It’s a very big universe, we should expect that understanding it will be a grand challenge.
That was an incredibly well written post. Thanks.
Don’t feed the troll, people.
I somewhat wonder if this idea might have a corollary in dealing with gauge invariance: in a number of theories in physics, there are ways to change the equations that have no impact whatsoever on the underlying physics. With basic, Newtonian gravity, for instance, you can add a constant number to the gravitational potential and nothing at all changes. Many theories have much more complicated ways of changing the math that leave everything about the physical behavior unchanged. In General Relativity, for instance, you can make any change in coordinates, and there is no change to the underlying behavior.
This becomes very important when performing sums over all possible configurations, such as one often does in statistical mechanics or when doing path integrals in quantum field theory: we have to be very careful not to sum up multiple configurations that are just the same thing related by a simple gauge transformation. If we do do this, we will get the wrong answer (often an infinite one), because we have counted up the same physical state many times.
So maybe this idea has an application here: after we’ve summed over all of the many worlds within a single cosmological horizon, we have already summed over all physical configurations, so attempting to sum over the configurations of neighboring regions of space-time would just be multiply-counting the same configuration all over again. And just like in gauge theory, it’s just not something you can do and get a reasonable answer.
I doubt there are multiple universes, I suspect consciousness collapses the wave function and does so because we and the universe are actually a simulation running on a computer. This solves the distinction between mind & matter, neither exist, ultimately all is information.
“Eventually it strays so far that it climbs right over the barrier in the direction of the true vacuum.”
I thought climbing was related to Hawking-Moss instanton and tunneling through the barrier Coleman-de Luccia.
Good food for thought .. Good thoughtexperiments .. Physical meditations ..
Thank you ..
What we see as the universe is in fact the multiverse. It’s the most probable outcome of probabilities. There are no other universes like ours, it wouldn’t make sense. The universe is the universe. There are no parallel worlds that are almost the same as ours, including ‘copies’ of ourselves. The universe is a game of infinities. The probability of differences vastly outnumbers the probability of similarity. Branching takes place at quantum mechanics level, contained within boundaries. In our daily world we don’t see any effects and everything seems logic and solid.
What we see as the universe is in fact a natural model in our brains. Light, colors, sound, senses, 3D depth, time, consciousness, logic, anything is basically a projection of our brains, in our brains. The universe itself may be totally different in nature as how we perceive it in daily life. The universe may be an infinite number of fractals without volume, size or dimensions, except within itself. And we happen to be part of that ‘super fractal’, are within that fractal, made of fractals. The universe is probably the outcome of simple mathematical laws. In a “nothing, nowhere and never” (as some people seem to look for as the origin of everything) mathematical laws would ‘still’ be valid, a true “nothing, nowhere and never” simply cannot exist. Existence by itself is a contradiction. To me it makes sense that there is ‘mostly nothing’ instead of absolutely nothing. What the basic nature of this something is? Nothing we can relate to in daily life. Infinite space maybe, or just infinity.
A Mandelbrot-like fractal is infinitely complex and has an infinite long boundary. Since the Mandelbrot lives within a small square on an infinite 2D plane the fractal cannot be ‘just’ 2D. It implies it has more than 2 dimensions.
3D fractals like the Mandelbox are fascinating. A simple function based on Mandelbrot projects an infinitely complex 3D structure with mathematical patterns and things that strongly resemble city landscapes, nature landscapes, microscopic images, coral, living creatures, art, architecture. Yet this Mandelbox fractal has no volume. It’s impossible, but if you were able to see the fractal after infinite iterations there wouldn’t be anything left. Calculated coordinates would have escaped the 3D coordinate space. Thin air so to speak. What you see depends on your position and zoom level. Basically, you only see something when you are ‘part’ or strongly related to the fractal. Explore the Mandelbox yourself with Mandelbulb3D. Maybe it’s a quite accurate but extremely simplified simulation of our universe.
“Rather, everything that you think might be going on outside can be encoded in the form of information on the horizon itself, just like for black holes!”
A big difference is that the interior of a black hole is finite, whereas the volume outside the cosmological horizon can be infinite, which could imply the encoding of an infinite amount of information. Discuss.
I find that appeal to a speculative idea (black hole complementarity) which may or may not be supported by an unsubstantiated hypothesis (string theory) is not a good way to build an argument in support of conflating two other speculative ideas (distinct, though related: bubble universes of eternal inflation and anthropic ensembles of cosmologies) with a third rather down-to-earth and reasonably well supported idea (MWI = no true collapse in QM).
Toppie,
That’s a great comment. I myself have toyed with the idea of the zero-worlds (as opposed to many worlds) hypothesis.
In fact, if someone more qualified than I could comment on this:
http://www.youtube.com/watch?v=dEaecUuEqfc
I’d be interested to see a debunking.
My personal feeling is that the organization of the brain constitutes a Turing machine, and as such, must construct the universe in a certain (Turing computable) way. Literally all information present in consciousness must be ordered this way. Our experiments increasingly show the quantum nature of matter exists at higher and higher masses, but the behavior is simply less relevant and apparent.
The insight, then, is that evolving a classical computer is much easier than a quantum one.
So apparently when I die, I don’t really die but just somebody observes me dead while someone else in a different space observes me still alive. And in the that different space when I die, I don’t really die…
So apparently there is life after death or life along with death or something like that.
Um, Geoff, my argument wasn’t that “since neither religion/god nor the multiverse has any evidence to support them, however religion is correct.” The fact is I didn’t make an argument for either. Nice try.
However, what I did mean to suggest, though, is that much of what now passes for science these days is (1) every bit as preposterous and bizarre as what is believed in most mainstream religions and (2) every bit as unverifiable, untestable, and empirically undocumented. Sometimes the numbers and data are even cooked and manipulated, as in the “science” of meteorology, our new state religion with Al Goreleone as its high priest.
The real point, sir, is that this would not otherwise deserve comment except that it’s your gang who generally ridicule people of faith, people who actually believe things far less bizarre and preposterous than much of what I read in these blogs and forums.
Incidentally, I happen not to belong to any organized religion, but I do believe in Intelligent Design. Where do you think all those beautiful equations and immutable, physical Laws you deal with came from? The tooth fairy?
I’m not a physicist, so I’m sure I’m out of my depth, but I have some thoughts.
In trying to reconcile this seeming duality between the ‘exterior’ inflationary multiverse and the ‘interior’ QM multiverse, shouldn’t we appeal to good old Occam’s Razor, which should suggest they’re identical? Developing the topology to make the two isomorphic would seem to be an interesting exploration. The non-orientable Möbius strip and Klein bottle, come to mind.
I think the holographic solution to unitarity is right. Still, the principle of complementarity has never meant anything more to me than the Heisenberg Uncertainity, so I’m skeptical by approaches that implicity elevate it to a status of fundamental law.
re: #37- Intelligent Design theory contradicts the theological axiom that god is perfect and created a perfect universe. One interpretation would have it that god found it impossible to make a seamlessly perfect world and left behind messy fingerprints on the work- tattletales if you will.
It also denies a basic and necessary characteristic of doing science at all- knowledge and understanding are cumulative over time (Newton stood on the shoulders of giants). One of the seminal proponents of ID theory reasoned that a complex bio-mechanical phenomenon could not have arisen naturally (at least he couldn’t figure it out) therefore it must be “artificial”. If that were true no one would ever be able to add to past knowledge and gain a better understanding of nature. Let us not call it a “sin” but rather the “error” of arrogance- “If I can’t do it, no one can!”.
As consolation, “Liberals are Evil” might contemplate that Hawking falls into a similar error when he came to the conclusion that it isn’t possible to “know the mind of God” and therefore it must be “turtles all the way down”- the Many Turtles Model if you will (though it’s not very nice to take consolation in the limitations of others- just your own.)
Sean, do you have any definite ideas about how this relates to your ideas on the arrow of time (which depend on eternal inflation), or is that still up in the air?
There may in fact be many Universes. Apparently though if they exist they are completely undetectable. Therefore it is meaningless and useless to even bother pondering such a thing. We should instead continue studying and working with our Universe to gain a better concept of how it works. As an alternative to Quantum Theory there is a new theory that describes and explains the mysteries of physical reality. While not disrespecting the value
of Quantum Mechanics as a tool to explain the role of quanta in our universe. This theory states that there is also a classical explanation for the paradoxes such as EPR and the Wave-Particle Duality. The Theory is called the Theory of Super Relativity and is located at: superrelativity. This theory is a philosophical attempt to reconnect the physical universe to
realism and deterministic concepts. It explains the mysterious.
Very prescient comments, Martin G; you’re a very, very bright man. You are quite right that ID contradicts the theolgies of most mainstream religions, but one can reconcile the notion of an Intelligent Designer with good and evil, or with an imperfect world, as it were.
A good start is by reflecting on the incontrovertible fact that nothing can exist (at least in this universe) without contemplating its mirror opposite. It’s only in this context that ANYTHING makes sense. For instance, good, or goodness, makes no sense at all as a behavorial model unless evil exists to give it meaning. Otherwise, it could not be said to be “good” in any meaningful sense. The same can be said for perfection as an abstract concept. It has no meaning unless we view the “flawed” in juxtaposition to perfection as an abstract notion..
There is something much deeper at play here, and perhaps we will all have to visit the other side, if there is one, to grasp what it’s all about. But thanks for your very wise and polite response.
What most people fail to appreciate is that black hole complementarity is actually one step weirder than quantum mechanics. In usual quantum mechanics, macroscopic observers will agree with each other whenever they compare their observations. The current distance separating them doesn’t matter because they can always meet up in the future. When we introduce general relativity however, there are some metrics where this can never happen and both observers can never compare notes. This gives us room for letting their observations disagree. This goes beyond what Bohr ever dreamed of.
In the Susskind and Bousso’s article, their complementarity is with respect to a supersymmetric terminal state with zero cosmological constant with no upper bound on its number of degrees of freedom.
If complementarity happens with respect to the causal patch horizon in the inflationary phase, with the stretched horizon at the apparent horizon, the holographic bound to the entropy of the causal patch will be smaller than the possible entropy of its child bubbles. Only entanglement entropy can solve this conundrum, and coarse graining over it will give rise to the second law of thermodynamics in the bubble.
Jesse, I don’t have any definite ideas, just some vague ones. There’s also some discussion in Nomura’s paper.
Can a wormhole cross an event horizon?
Pingback: WPS | Web Picks Sceptique for May 27, 2011 « The Call of Troythulu – Musings of a Skeptophrenic
There’s a simpler way. According to QM, everything that can happen at a place, does happen, in superposition. If the laws of physics are the same throughout the universe, and the initial state was uniform (translational symmetry) then everything that can happen does happen everywhere, and the wavefunction is identical at every point.
That this includes false vacua is just one special case. You and your entire history are another possible quantum event, that therefore occurs everywhere simultaneously. Although it is difficult to work out what it means to talk about a definite point in space in a relativistic, translationally symmetric universe…
To answer #4 on where the mass comes from – the answer is that it doesn’t, because the universe doesn’t really split. It’s just an analogy to explain the concept in everyday language. If you think about a single electron as it passes through a pair of splits, does the electron passing through one slit see itself passing through the other? The different possible paths the electron can take are the different “worlds” of the MWI. A wavefunction spread out across space is a superposition of many instances of a single particle each at a single place, surrounded as far as it can tell by empty space. Components of the wavefunction for a single particle don’t ‘interact’ (for example, a charged particle wavefunction doesn’t repel itself electrostatically), in the same sort of way that ripples on a pond pass through one another without affecting one another. It’s all still one universe, but bits of it act independently, as if the rest of it didn’t exist.
“Many Worlds” is really just a bad metaphor for a very routine bit of quantum mechanics that has already been accepted by anyone who accepts that the electron really does – in some sense – go through both slits at once.
# 48 Nullius in Verba — The electron does not – in any sense – go through both slits at once. The electron goes through a single slit. However, its path is influenced by the field disturbance it creates by its motion. The field disturbance takes the same interference form in passing through the slits as a light wave. So a series of electrons — which can be separated not only in time but also in space so long as the equipment is identical — will over many impacts build up an interference pattern, so long as the possibility of interference is not destroyed by a detector. But the pattern is due to the electron influencing its own path, not to the electron going through both slits at once.
#49,
That sounds like the DeBroglie-Bohm ‘pilot wave’ interpretation. It’s non-local in its handling of EPR-type situations, and MWI would say the particle part was superfluous given that the pilot wave does everything the MWI says the wavefunction does, but as an interpretation of QM has exactly the same observational predictions as all the others.
I can’t tell you which interpretation of QM is “correct” – it’s arguably an unscientific question. (Depending on how you evaluate issues like parsimony, aesthetics, and explanatory power in science.) But what I was talking about above was what the claims of the Many Worlds interpretation were, not whether it was true.