The Screwy Universe

I’ve been meaning to post about the claim that experimenters have demonstrated that the proton/electron mass ratio is changing with time. Although it’s a fascinating discovery if true, there’s something that doesn’t quite smell right about it. So I hit on the idea of first posting about the idea of physics claims not “smelling” right more generally. But then I thought that such a post would necessarily involve a careful exposition of one particular example. So it’s time for the story of the Screwy Universe.

In April 1997, while a postdoc at the Institute for Theoretical Physics at UC Santa Barbara, I received an email from George Field, who had been my Ph.D. advisor. He was suggesting that I take a look at a news article that had appeared on the front page of the New York Times. George is one of my favorite people in the whole world, and I owe whatever success I may have had as a scientist to his insightful guidance in my early career. But okay, I was busy, and didn’t immediately look at the article — lots of crazy stuff appears in the NYT, after all.

But George wrote again, gently suggesting that I really should take a look at this article, which I finally did. And it was indeed striking. Two scientists, Borge Nodland of the University of Rochester and John Ralston of the University of Kansas, were claiming that they had detected a violation of a fundamental principle of modern cosmology — isotropy, the idea that space looks the same in every direction. In particular, they had considered the polarization of radio waves coming from distant quasars, and looked for a rotation of the polarization angle as the waves traveled through space. And they had found evidence of just such a rotation! If N&R were right, there was a preferred direction in the cosmos — along that direction, polarized radio waves would gently corkscrew as they traveled through space, while in the opposite direction they would twist the other way. Completely contrary, of course, to our conventional expectations, which are that (1) polarized waves maintain their polarization angles in empty space, rather than rotating, and (2) every direction in the sky is basically equivalent to every other direction.

Clearly important stuff. But for George and me this hit particularly close to home, as we had previously collaborated with particle theorist Roman Jackiw on a very similar-sounding project, looking for gentle rotations in the polarization of distant sources (and not finding any). In fact, this work with George and Roman was the topic of my first published paper. Our motivation was to test Lorentz invariance by searching for the effects of a constant vector field spread throughout spacetime. It turns out that such a vector can couple to ordinary electromagnetism, but only in certain specified ways. We showed that, if the vector pointed mostly in the time direction of spacetime, its effect would be to uniformly rotate the observed polarization of distant radio sources; we then searched for such an effect in the existing data, and didn’t find any. My job as the beginning graduate student was to look in the literature for measurements of the polarization angles and redshifts of as many galaxies as I could find. I managed to scrape up 160 such galaxies, which was enough to put a good limit on the effect we were looking for. (I should say that, as a nervous beginning graduate student, George was extremely intimidating because of his formidable intellect and amazing accomplishments, but in other circumstances one would recognize that he was extremely gentle and easygoing. Roman, on the other hand, was intimidating, period. But also fantastically smart, and an excellent collaborator once one calmed down and got into the science.)

At the time, anxious young ingenue that I was, I was somewhat worried that writing my first paper on a topic as outlandish as Lorentz violation might spell the premature end of my career. Nowadays, of course, it is all the rage, and we are proud pioneers.

So the news of Nodand and Ralston’s work had a personal resonance — it sounded like they were investigating something similar. And then I noticed in the NYT story — 160 radio galaxies! These guys were using the very data I had typed in as a first-year grad student. (Although, as it later turned out, they were careful enough to check everything, and had found a few typos.) In fact they had basically done exactly the same thing that we had done, except that they had considered a Lorentz-violating vector field that was pointing in a spatial direction instead of in the time direction. As a result, they were asking whether there was a direction-dependent rotation of polarizations — clockwise if you looked at one side of the sky, counter-clockwise if you looked at the other — rather than a uniform one across the sky. And, remarkably, they seemed to be saying that there was such a rotation!

But I didn’t believe it, not for a second. True, we hadn’t carefully placed a limit on such an effect, but I was convinced that I would have noticed it in the course of playing around with the data. Not to mention, there was no good theoretical reason to suspect that such an effect might exist. In short, it didn’t smell right.

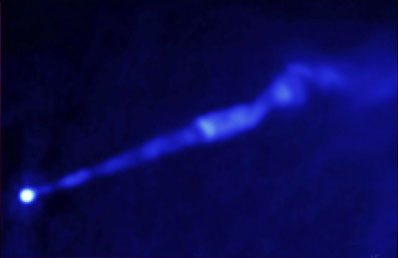
As it turns out, Nodland and Ralston had simply made a mistake. What they, and before them we, were doing was comparing the polarization from distant quasars to their orientation angles on the sky. Many of these quasars have extended jets coming from massive black holes at their centers, and the orientation of these jets defines (more or less) an orientation on the sky, as in the image of M87 above from the Very Large Array. We expect that there will be magnetic fields that stretch along the jet, and that free electrons will be spinning in circles perpendicular to the magnetic fields. These electrons then emit synchrotron radiation — the motion of the electrons produces a time-dependent electric field, which travels to us as radio waves. Because that motion is perpendicular to the background magnetic field (and thus to the jet), the electric field in the resulting radio waves is likewise perpendicular to the jet, and its that electric field that defines the polarization. In other words, on physical grounds we anticipate that the quasar polarization is at roughly 90 degrees to the angle of the jet on the sky. We were looking for any systematic deviations from this relationship, either in the same sense everywhere (George, Roman and I) or clockwise in one direction and counterclockwise in the other (Nodland and Ralston).

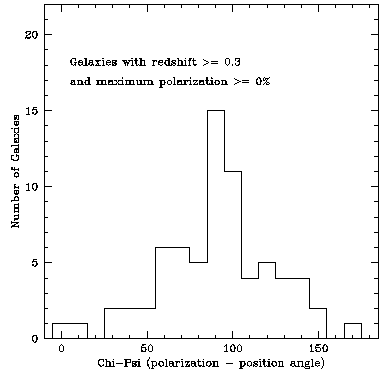
You’ll notice, though, that neither the polarization angle nor the orientation angle of the jet is uniquely defined; rotating by 180 degrees gives you an equally good answer. So the difference between them is likewise subject to a 180-degree ambiguity. Since you expect the difference to be 90 degrees, this doesn’t make much of a difference in practice; indeed, if you resolve the ambiguity by defining the difference to lie between 0 and 180 degrees, there is an obvious peak at 90.

However, Nodland and Ralston were working under the assumption that the difference should naturally be zero degrees, rather than 90. That is, they assumed that the polarization and orientation should naturally be parallel. To resolve the 180-degree ambiguity, they defined the difference between the polarization and orientation angles to be between 0 and 180 degrees if the quasar was in one hemisphere of the sky, and between -180 degrees and zero if it was in the opposite hemisphere. This was a bad thing to do, especially when combined with what they did next, which was to then plot the data as a function of redshift and fit it to a straight line. In other words, they made a plot in which all of the points would lie in the bottom left and top right quadrants by hypothesis, and then asked if the best-fit line would have a nonzero slope (which would purportedly indicate that the polarization had been rotating as it traveled through space). Of course, it had to, by construction.

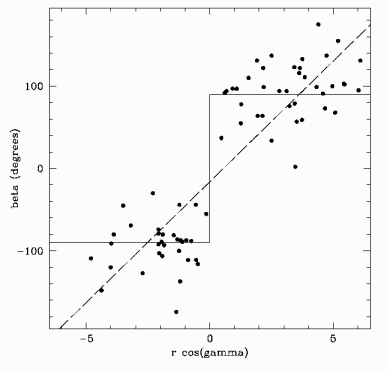
Here are the data, with the diagonal N&R fit. The piecewise-constant line, just indicating that the data are clustered at plus or minus 90 degrees, is statistically a much better fit. Indeed, if N&R had been correct, the prominent peak at 90 degrees in the above histogram would had to have been a complete accident, representing a bunch of points really centered around zero that had fortuitously been rotated to plus or minus 90. Much easier to believe that the polarization is perpendicular to the jets, as it should be, and that there is no fundamental anisotropy or preferred direction in the universe.

Of course, appearing on the front page of the NYT, the N&R paper sparked a great deal of interest and follow-up papers, as detailed here (with many by-now-broken links). Even an appearance in the funny pages! George and I wrote a quick response paper, one of several that appeared. It was the only time in my life when I worked feverishly over the course of a couple of days to write a paper from scratch — not normally my favored mode of operation.

But the good news is that the dust-up put this anisotropy stuff back in my mind, and a year later I realized that you could get exactly this kind of effect — a rotation of polarized photons as they traveled from distant quasars to us — from quintessence, a dynamical field that could be the dark energy. An evolving scalar field naturally violates Lorentz invariance by picking out a preferred direction in spacetime — in this case, the direction in which the field is evolving (presumably the timelike one). Models of quintessence that feature symmetries to protect them from being ruled out tend to allow for only one kind of interaction with ordinary matter, which is exactly the kind that can push around the polarization states of photons. Interestingly, the current quasar data are not quite good enough to test this prediction at sufficient precision that we should have seen the effect if it is really there. So there is one way to win the Nobel Prize lurking out there — directly detect the existence of quintessence by discovering a systematic redshift-dependent rotation of polarized light from distant galaxies. You only have to improve the existing limits by one or two orders of magnitude. So what are you waiting for?